בעיה באיש מכירות נסיעות

בקטע הזה מופיעה דוגמה שמראה איך לפתור את הבעיה באיש הנסיעות (TSP) של המיקומים המוצגים במפה הבאה.

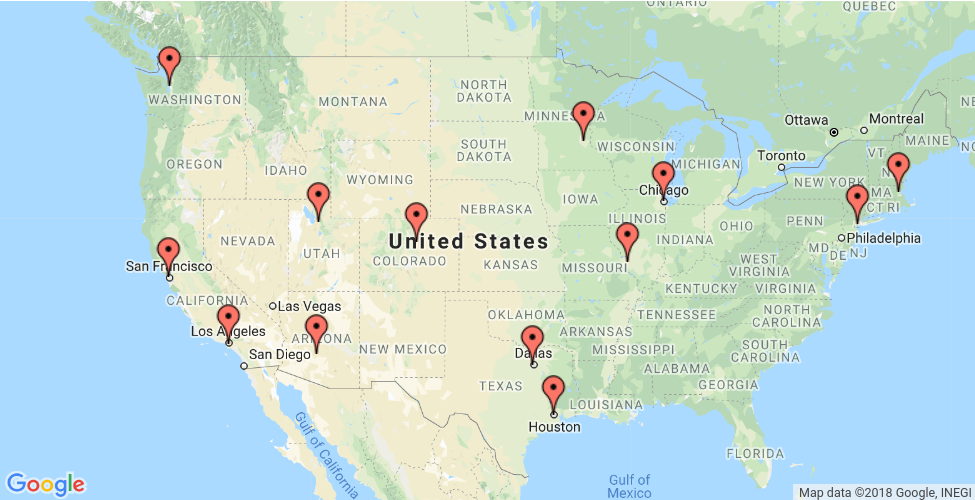
בקטעים הבאים מוצגות תוכניות ב-Python , C++ , Java ו-C# שפותרות את ה-TSP באמצעות OR-Tools

יצירת הנתונים

הקוד הבא יוצר את הנתונים עבור הבעיה.

Python

def create_data_model():
    """Stores the data for the problem."""
    data = {}
    data["distance_matrix"] = [
        [0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972],
        [2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579],
        [713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260],
        [1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987],
        [1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371],
        [1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999],
        [2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701],
        [213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099],
        [2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600],
        [875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162],
        [1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200],
        [2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504],
        [1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0],
    ]
    data["num_vehicles"] = 1
    data["depot"] = 0
    return data

C++‎

struct DataModel {
  const std::vector<std::vector<int64_t>> distance_matrix{
      {0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972},
      {2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579},
      {713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260},
      {1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987},
      {1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371},
      {1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999},
      {2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701},
      {213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099},
      {2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600},
      {875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162},
      {1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200},
      {2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504},
      {1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0},
  };
  const int num_vehicles = 1;
  const RoutingIndexManager::NodeIndex depot{0};
};

Java

static class DataModel {
  public final long[][] distanceMatrix = {
      {0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972},
      {2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579},
      {713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260},
      {1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987},
      {1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371},
      {1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999},
      {2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701},
      {213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099},
      {2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600},
      {875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162},
      {1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200},
      {2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504},
      {1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0},
  };
  public final int vehicleNumber = 1;
  public final int depot = 0;
}

C#‎

class DataModel
{
    public long[,] DistanceMatrix = {
        { 0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972 },
        { 2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579 },
        { 713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260 },
        { 1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987 },
        { 1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371 },
        { 1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999 },
        { 2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701 },
        { 213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099 },
        { 2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600 },
        { 875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162 },
        { 1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200 },
        { 2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504 },
        { 1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0 },
    };
    public int VehicleNumber = 1;
    public int Depot = 0;
};

מטריצת המרחק היא מערך שהפונקציה i שלו, j היא המרחק מהמיקום i למיקום j במיילים, שבו אינדקסי המערך תואמים למיקומים לפי הסדר הבא:

0. New York - 1. Los Angeles - 2. Chicago - 3. Minneapolis - 4. Denver - 5. Dallas
- 6. Seattle - 7. Boston - 8. San Francisco - 9. St. Louis - 10. Houston - 11. Phoenix - 12. Salt Lake City

הערה: סדר המיקומים במטריצת המרחק הוא שרירותי ואינו קשור לסדר המיקומים בכל פתרון ב-TSP.

בנוסף, הנתונים כוללים:

• מספר כלי הרכב בבעיה, שהוא 1 כי מדובר ב-TSP. (אם מדובר בבעיה בניתוב (VRP), מספר כלי הרכב יכול להיות גדול מ-1.)
• Depot: נקודת ההתחלה והסיום של המסלול. במקרה הזה, המחסן הוא 0, בהתאמה לניו יורק.

הערה: מאחר שפותר הבעיות בניתוב מבצע את כל החישובים עם מספרים שלמים, הקריאה החוזרת (callback) של המרחק חייבת להחזיר את המספר השלם של שני המיקומים. אם הערכים ב-data['distance_matrix'] הם לא מספרים שלמים, עליכם לעגל את ערכי המטריצה או את ערכי ההחזרה של הקריאה החוזרת למספרים שלמים. לדוגמה, שינוי מטריצת המרחק מאפשרת לראות איך להימנע מבעיות שנגרמו עקב שגיאת עיגול.

דרכים נוספות ליצירת מטריצת מרחקים

בדוגמה הזו, מטריצת המרחק מוגדרת באופן מפורש בתוכנית. אפשר גם להשתמש בפונקציה כדי לחשב מרחקים בין מיקומים: לדוגמה, הנוסחה האוקלידית לציון המרחק בין נקודות במטוס. עם זאת, עכשיו יעיל יותר לחשב מראש את כל המרחקים בין המיקומים ולאחסן אותם במטריצה, במקום לחשב אותם בזמן הריצה. במאמר דוגמה: תרגול קידוח מעגלי לדוגמה ליצירת מטריצת המרחק כך.

אפשרות נוספת היא להשתמש ב-API של מפות Google למטריצת מרחקים כדי ליצור באופן דינמי מטריצת מרחק (או זמן נסיעה) לבעיה בניתוב.

יצירת מודל הניתוב

הקוד הבא בחלק הראשי של התוכניות יוצר את מנהל האינדקס (manager) ואת מודל הניתוב (routing). השיטה manager.IndexToNode ממירה את האינדקסים הפנימיים של המפענח (שאפשר להתעלם מהם) למספרים של המיקומים. מספרי המיקום תואמים לאינדקסים של מטריצת המרחק.

Python

data = create_data_model()
manager = pywrapcp.RoutingIndexManager(
    len(data["distance_matrix"]), data["num_vehicles"], data["depot"]
)
routing = pywrapcp.RoutingModel(manager)

C++‎

DataModel data;
RoutingIndexManager manager(data.distance_matrix.size(), data.num_vehicles,
                            data.depot);
RoutingModel routing(manager);

Java

final DataModel data = new DataModel();
RoutingIndexManager manager =
    new RoutingIndexManager(data.distanceMatrix.length, data.vehicleNumber, data.depot);
RoutingModel routing = new RoutingModel(manager);

C#‎

DataModel data = new DataModel();
RoutingIndexManager manager =
    new RoutingIndexManager(data.DistanceMatrix.GetLength(0), data.VehicleNumber, data.Depot);
RoutingModel routing = new RoutingModel(manager);

הקלט עבור RoutingIndexManager הוא:

• מספר השורות של מטריצת המרחק, שהיא מספר המיקומים (כולל המאגר).
• מספר הרכבים שנתקלו בבעיה.
• את הצומת המתאים למאגר.

הערה: בבעיות אחרות אפשר לציין מיקומים שונים של נקודות התחלה וסיום לנתיבים. לשם כך, עליכם להעביר שני וקטורים, הראשון המכיל את מיקומי ההתחלה והשני המכיל את מיקומי הסיום, במקום depot יחיד. לדוגמה, תוכלו להגדיר את מיקומי ההתחלה והסיום של המסלולים.

יצירת קריאה חוזרת (callback) מרחוק

כדי להשתמש בפותר הניתוב, עליך ליצור קריאה חוזרת (callback) של מרחקים (או תחבורה ציבורית): פונקציה שמקבלת צמד של מיקומים ומחזירה את המרחק ביניהם. הדרך הקלה ביותר לעשות זאת היא להשתמש במטריצת המרחק.

הפונקציה הבאה יוצרת את הקריאה החוזרת (callback) ומתעדת אותה עם פותר הבעיות בתור transit_callback_index.

Python

def distance_callback(from_index, to_index):
    """Returns the distance between the two nodes."""
    # Convert from routing variable Index to distance matrix NodeIndex.
    from_node = manager.IndexToNode(from_index)
    to_node = manager.IndexToNode(to_index)
    return data["distance_matrix"][from_node][to_node]

transit_callback_index = routing.RegisterTransitCallback(distance_callback)
  

C++

const int transit_callback_index = routing.RegisterTransitCallback(
    [&data, &manager](const int64_t from_index,
                      const int64_t to_index) -> int64_t {
      // Convert from routing variable Index to distance matrix NodeIndex.
      const int from_node = manager.IndexToNode(from_index).value();
      const int to_node = manager.IndexToNode(to_index).value();
      return data.distance_matrix[from_node][to_node];
    });
  

Java

final int transitCallbackIndex =
    routing.registerTransitCallback((long fromIndex, long toIndex) -> {
      // Convert from routing variable Index to user NodeIndex.
      int fromNode = manager.indexToNode(fromIndex);
      int toNode = manager.indexToNode(toIndex);
      return data.distanceMatrix[fromNode][toNode];
    });
  

C#

int transitCallbackIndex = routing.RegisterTransitCallback((long fromIndex, long toIndex) =>
                                                           {
                                                               // Convert from routing variable Index to
                                                               // distance matrix NodeIndex.
                                                               var fromNode = manager.IndexToNode(fromIndex);
                                                               var toNode = manager.IndexToNode(toIndex);
                                                               return data.DistanceMatrix[fromNode, toNode];
                                                           });
  

The callback accepts two indices, from_index and to_index, and returns the corresponding entry of the distance matrix.

Set the cost of travel

The arc cost evaluator tells the solver how to calculate the cost of travel between any two locations — in other words, the cost of the edge (or arc) joining them in the graph for the problem. The following code sets the arc cost evaluator.

Python

routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index)

C++‎

routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index);

Java

routing.setArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

C#‎

routing.SetArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

בדוגמה הזו, מעריך העלויות של קשת הוא transit_callback_index, שמהווה את האזכור הפנימי של רכיב הקריאה החוזרת (callback) של הלקוח/ה. כלומר, עלות הנסיעה בין שני המיקומים היא המרחק שמופיע ביניהם. עם זאת, באופן כללי, העלויות עשויות לכלול גם גורמים אחרים.

בנוסף, באמצעות המאפיין routing.SetArcCostEvaluatorOfVehicle() אפשר להגדיר כמה פונקציות של קשתות עלות שתלויות בסוג הרכב. לדוגמה, אם כלי הרכב שונים במהירויות שונות, אפשר להגדיר את עלות הנסיעה בין מיקומים כך שהם יהיו חלקי המרחק של מהירות הרכב, כלומר את זמן הנסיעה.

הגדרת פרמטרים של חיפוש

הקוד הבא מגדיר את פרמטרי החיפוש שמוגדרים כברירת מחדל ושיטה היאוריסטית למציאת הפתרון הראשון:

Python

search_parameters = pywrapcp.DefaultRoutingSearchParameters()
search_parameters.first_solution_strategy = (
    routing_enums_pb2.FirstSolutionStrategy.PATH_CHEAPEST_ARC
)

C++‎

RoutingSearchParameters searchParameters = DefaultRoutingSearchParameters();
searchParameters.set_first_solution_strategy(
    FirstSolutionStrategy::PATH_CHEAPEST_ARC);

Java

RoutingSearchParameters searchParameters =
    main.defaultRoutingSearchParameters()
        .toBuilder()
        .setFirstSolutionStrategy(FirstSolutionStrategy.Value.PATH_CHEAPEST_ARC)
        .build();

C#‎

RoutingSearchParameters searchParameters =
    operations_research_constraint_solver.DefaultRoutingSearchParameters();
searchParameters.FirstSolutionStrategy = FirstSolutionStrategy.Types.Value.PathCheapestArc;

הקוד מגדיר את אסטרטגיית הפתרון הראשונה כ-PATH_CHEAPEST_ARC, וכך נוצר נתיב ראשוני לפותר על ידי הוספה חוזרת של קצוות עם משקל מזערי שלא מובילים לצומת שבו ביקרתם קודם (חוץ מהמחסן). לאפשרויות נוספות, קראו את המאמר שיטת פתרון ראשונה.

הוספת מדפסת הפתרון

הפונקציה שמציגה את הפתרון המוזכר מופיעה בהמשך. הפונקציה מחלצת את המסלול מהפתרון ומדפיסה אותו במסוף.

Python

def print_solution(manager, routing, solution):
    """Prints solution on console."""
    print(f"Objective: {solution.ObjectiveValue()} miles")
    index = routing.Start(0)
    plan_output = "Route for vehicle 0:\n"
    route_distance = 0
    while not routing.IsEnd(index):
        plan_output += f" {manager.IndexToNode(index)} ->"
        previous_index = index
        index = solution.Value(routing.NextVar(index))
        route_distance += routing.GetArcCostForVehicle(previous_index, index, 0)
    plan_output += f" {manager.IndexToNode(index)}\n"
    print(plan_output)
    plan_output += f"Route distance: {route_distance}miles\n"

C++‎

//! @brief Print the solution.
//! @param[in] manager Index manager used.
//! @param[in] routing Routing solver used.
//! @param[in] solution Solution found by the solver.
void PrintSolution(const RoutingIndexManager& manager,
                   const RoutingModel& routing, const Assignment& solution) {
  // Inspect solution.
  LOG(INFO) << "Objective: " << solution.ObjectiveValue() << " miles";
  int64_t index = routing.Start(0);
  LOG(INFO) << "Route:";
  int64_t distance{0};
  std::stringstream route;
  while (!routing.IsEnd(index)) {
    route << manager.IndexToNode(index).value() << " -> ";
    const int64_t previous_index = index;
    index = solution.Value(routing.NextVar(index));
    distance += routing.GetArcCostForVehicle(previous_index, index, int64_t{0});
  }
  LOG(INFO) << route.str() << manager.IndexToNode(index).value();
  LOG(INFO) << "Route distance: " << distance << "miles";
  LOG(INFO) << "";
  LOG(INFO) << "Advanced usage:";
  LOG(INFO) << "Problem solved in " << routing.solver()->wall_time() << "ms";
}

Java

/// @brief Print the solution.
static void printSolution(
    RoutingModel routing, RoutingIndexManager manager, Assignment solution) {
  // Solution cost.
  logger.info("Objective: " + solution.objectiveValue() + "miles");
  // Inspect solution.
  logger.info("Route:");
  long routeDistance = 0;
  String route = "";
  long index = routing.start(0);
  while (!routing.isEnd(index)) {
    route += manager.indexToNode(index) + " -> ";
    long previousIndex = index;
    index = solution.value(routing.nextVar(index));
    routeDistance += routing.getArcCostForVehicle(previousIndex, index, 0);
  }
  route += manager.indexToNode(routing.end(0));
  logger.info(route);
  logger.info("Route distance: " + routeDistance + "miles");
}

C#‎

/// <summary>
///   Print the solution.
/// </summary>
static void PrintSolution(in RoutingModel routing, in RoutingIndexManager manager, in Assignment solution)
{
    Console.WriteLine("Objective: {0} miles", solution.ObjectiveValue());
    // Inspect solution.
    Console.WriteLine("Route:");
    long routeDistance = 0;
    var index = routing.Start(0);
    while (routing.IsEnd(index) == false)
    {
        Console.Write("{0} -> ", manager.IndexToNode((int)index));
        var previousIndex = index;
        index = solution.Value(routing.NextVar(index));
        routeDistance += routing.GetArcCostForVehicle(previousIndex, index, 0);
    }
    Console.WriteLine("{0}", manager.IndexToNode((int)index));
    Console.WriteLine("Route distance: {0}miles", routeDistance);
}

הפונקציה מציגה את המסלול האופטימלי ואת המרחק שלו, שנקבע על ידי ObjectiveValue().

פתרון והדפסה של הפתרון

לבסוף, אפשר להתקשר לפותר הבעיות ולהדפיס את הפתרון:

Python

solution = routing.SolveWithParameters(search_parameters)
if solution:
    print_solution(manager, routing, solution)

C++‎

const Assignment* solution = routing.SolveWithParameters(searchParameters);
PrintSolution(manager, routing, *solution);

Java

Assignment solution = routing.solveWithParameters(searchParameters);
printSolution(routing, manager, solution);

C#‎

Assignment solution = routing.SolveWithParameters(searchParameters);
PrintSolution(routing, manager, solution);

הפונקציה הזו מחזירה את הפתרון ומציגה את המסלול האופטימלי.

הפעלת התוכניות

כשמפעילים את התוכניות, מוצג הפלט הבא.

Objective: 7293 miles
Route for vehicle 0:
 0 -> 7 -> 2 -> 3 -> 4 -> 12 -> 6 -> 8 -> 1 -> 11 -> 10 -> 5 -> 9 -> 0

בדוגמה הזו, יש רק מסלול אחד מפני שהוא TSP. אבל בבעיות כלליות יותר של ניתוב רכב, הפתרון מכיל מספר מסלולים.

שמירת מסלולים לרשימה או למערך

כחלופה להדפסת הפתרון ישירות, אפשר לשמור את המסלול (או מסלולים, ל-VRP) ברשימה או במערך. זה מאפשר לכם להפוך את המסלולים לזמינים במקרה שתרצו לעשות איתם משהו. לדוגמה, אפשר להפעיל את התוכנית מספר פעמים באמצעות פרמטרים שונים, ולשמור בקובץ את המסלולים שנמצאו בפתרונות שהוחזרו.

הפונקציות הבאות שומרות את המסלולים שבפתרון לכל VRP (אולי עם מספר כלי רכב) כרשימה (Python) או כמערך (C++ ).

Python

def get_routes(solution, routing, manager):
  """Get vehicle routes from a solution and store them in an array."""
  # Get vehicle routes and store them in a two dimensional array whose
  # i,j entry is the jth location visited by vehicle i along its route.
  routes = []
  for route_nbr in range(routing.vehicles()):
    index = routing.Start(route_nbr)
    route = [manager.IndexToNode(index)]
    while not routing.IsEnd(index):
      index = solution.Value(routing.NextVar(index))
      route.append(manager.IndexToNode(index))
    routes.append(route)
  return routes

C++‎

std::vector<std::vector<int>> GetRoutes(const Assignment& solution,
                                        const RoutingModel& routing,
                                        const RoutingIndexManager& manager) {
  // Get vehicle routes and store them in a two dimensional array, whose
  // i, j entry is the node for the jth visit of vehicle i.
  std::vector<std::vector<int>> routes(manager.num_vehicles());
  // Get routes.
  for (int vehicle_id = 0; vehicle_id < manager.num_vehicles(); ++vehicle_id) {
    int64_t index = routing.Start(vehicle_id);
    routes[vehicle_id].push_back(manager.IndexToNode(index).value());
    while (!routing.IsEnd(index)) {
      index = solution.Value(routing.NextVar(index));
      routes[vehicle_id].push_back(manager.IndexToNode(index).value());
    }
  }
  return routes;
}

אפשר להשתמש בפונקציות האלה כדי לקבל את המסלולים בכל אחת מהדוגמאות של VRP בקטע 'ניתוב'.

הקוד הבא מציג את המסלולים.

Python

routes = get_routes(solution, routing, manager)
# Display the routes.
for i, route in enumerate(routes):
  print('Route', i, route)

C++‎

const std::vector⟨std::vector⟨int⟩⟩
    routes = GetRoutes(*solution,
                        routing,
                        manager);
// Display the routes.
for (int vehicle_id = 0; vehicle_id < routes.size(); ++vehicle_id) {
  LOG(INFO) << "Route " << vehicle_id;
  for (int j = 1; j < routes[vehicle_id].size(); ++j) {
    LOG(INFO) << routes[vehicle_id][j];
  }
}

בדוגמה הנוכחית, הקוד הזה מחזיר את הנתיב הבא:

Route 0 [0, 7, 2, 3, 4, 12, 6, 8, 1, 11, 10, 5, 9, 0]

כתרגיל, משנים את הקוד שלמעלה כדי לעצב את הפלט באותו אופן כמו מדפסת הפתרון של התוכנית.

השלמת התוכניות

תוכניות ה-TSP המלאות מוצגות בהמשך.

Python

"""Simple Travelling Salesperson Problem (TSP) between cities."""

from ortools.constraint_solver import routing_enums_pb2
from ortools.constraint_solver import pywrapcp


def create_data_model():
    """Stores the data for the problem."""
    data = {}
    data["distance_matrix"] = [
        [0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972],
        [2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579],
        [713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260],
        [1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987],
        [1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371],
        [1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999],
        [2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701],
        [213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099],
        [2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600],
        [875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162],
        [1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200],
        [2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504],
        [1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0],
    ]
    data["num_vehicles"] = 1
    data["depot"] = 0
    return data


def print_solution(manager, routing, solution):
    """Prints solution on console."""
    print(f"Objective: {solution.ObjectiveValue()} miles")
    index = routing.Start(0)
    plan_output = "Route for vehicle 0:\n"
    route_distance = 0
    while not routing.IsEnd(index):
        plan_output += f" {manager.IndexToNode(index)} ->"
        previous_index = index
        index = solution.Value(routing.NextVar(index))
        route_distance += routing.GetArcCostForVehicle(previous_index, index, 0)
    plan_output += f" {manager.IndexToNode(index)}\n"
    print(plan_output)
    plan_output += f"Route distance: {route_distance}miles\n"


def main():
    """Entry point of the program."""
    # Instantiate the data problem.
    data = create_data_model()

    # Create the routing index manager.
    manager = pywrapcp.RoutingIndexManager(
        len(data["distance_matrix"]), data["num_vehicles"], data["depot"]
    )

    # Create Routing Model.
    routing = pywrapcp.RoutingModel(manager)


    def distance_callback(from_index, to_index):
        """Returns the distance between the two nodes."""
        # Convert from routing variable Index to distance matrix NodeIndex.
        from_node = manager.IndexToNode(from_index)
        to_node = manager.IndexToNode(to_index)
        return data["distance_matrix"][from_node][to_node]

    transit_callback_index = routing.RegisterTransitCallback(distance_callback)

    # Define cost of each arc.
    routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index)

    # Setting first solution heuristic.
    search_parameters = pywrapcp.DefaultRoutingSearchParameters()
    search_parameters.first_solution_strategy = (
        routing_enums_pb2.FirstSolutionStrategy.PATH_CHEAPEST_ARC
    )

    # Solve the problem.
    solution = routing.SolveWithParameters(search_parameters)

    # Print solution on console.
    if solution:
        print_solution(manager, routing, solution)


if __name__ == "__main__":
    main()

C++‎

#include <cmath>
#include <cstdint>
#include <sstream>
#include <vector>

#include "ortools/constraint_solver/routing.h"
#include "ortools/constraint_solver/routing_enums.pb.h"
#include "ortools/constraint_solver/routing_index_manager.h"
#include "ortools/constraint_solver/routing_parameters.h"

namespace operations_research {
struct DataModel {
  const std::vector<std::vector<int64_t>> distance_matrix{
      {0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972},
      {2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579},
      {713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260},
      {1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987},
      {1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371},
      {1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999},
      {2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701},
      {213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099},
      {2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600},
      {875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162},
      {1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200},
      {2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504},
      {1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0},
  };
  const int num_vehicles = 1;
  const RoutingIndexManager::NodeIndex depot{0};
};

//! @brief Print the solution.
//! @param[in] manager Index manager used.
//! @param[in] routing Routing solver used.
//! @param[in] solution Solution found by the solver.
void PrintSolution(const RoutingIndexManager& manager,
                   const RoutingModel& routing, const Assignment& solution) {
  // Inspect solution.
  LOG(INFO) << "Objective: " << solution.ObjectiveValue() << " miles";
  int64_t index = routing.Start(0);
  LOG(INFO) << "Route:";
  int64_t distance{0};
  std::stringstream route;
  while (!routing.IsEnd(index)) {
    route << manager.IndexToNode(index).value() << " -> ";
    const int64_t previous_index = index;
    index = solution.Value(routing.NextVar(index));
    distance += routing.GetArcCostForVehicle(previous_index, index, int64_t{0});
  }
  LOG(INFO) << route.str() << manager.IndexToNode(index).value();
  LOG(INFO) << "Route distance: " << distance << "miles";
  LOG(INFO) << "";
  LOG(INFO) << "Advanced usage:";
  LOG(INFO) << "Problem solved in " << routing.solver()->wall_time() << "ms";
}

void Tsp() {
  // Instantiate the data problem.
  DataModel data;

  // Create Routing Index Manager
  RoutingIndexManager manager(data.distance_matrix.size(), data.num_vehicles,
                              data.depot);

  // Create Routing Model.
  RoutingModel routing(manager);

  const int transit_callback_index = routing.RegisterTransitCallback(
      [&data, &manager](const int64_t from_index,
                        const int64_t to_index) -> int64_t {
        // Convert from routing variable Index to distance matrix NodeIndex.
        const int from_node = manager.IndexToNode(from_index).value();
        const int to_node = manager.IndexToNode(to_index).value();
        return data.distance_matrix[from_node][to_node];
      });

  // Define cost of each arc.
  routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index);

  // Setting first solution heuristic.
  RoutingSearchParameters searchParameters = DefaultRoutingSearchParameters();
  searchParameters.set_first_solution_strategy(
      FirstSolutionStrategy::PATH_CHEAPEST_ARC);

  // Solve the problem.
  const Assignment* solution = routing.SolveWithParameters(searchParameters);

  // Print solution on console.
  PrintSolution(manager, routing, *solution);
}

}  // namespace operations_research

int main(int /*argc*/, char* /*argv*/[]) {
  operations_research::Tsp();
  return EXIT_SUCCESS;
}

Java

package com.google.ortools.constraintsolver.samples;
import com.google.ortools.Loader;
import com.google.ortools.constraintsolver.Assignment;
import com.google.ortools.constraintsolver.FirstSolutionStrategy;
import com.google.ortools.constraintsolver.RoutingIndexManager;
import com.google.ortools.constraintsolver.RoutingModel;
import com.google.ortools.constraintsolver.RoutingSearchParameters;
import com.google.ortools.constraintsolver.main;
import java.util.logging.Logger;


/** Minimal TSP using distance matrix. */
public class TspCities {
  private static final Logger logger = Logger.getLogger(TspCities.class.getName());

  static class DataModel {
    public final long[][] distanceMatrix = {
        {0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972},
        {2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579},
        {713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260},
        {1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987},
        {1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371},
        {1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999},
        {2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701},
        {213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099},
        {2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600},
        {875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162},
        {1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200},
        {2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504},
        {1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0},
    };
    public final int vehicleNumber = 1;
    public final int depot = 0;
  }

  /// @brief Print the solution.
  static void printSolution(
      RoutingModel routing, RoutingIndexManager manager, Assignment solution) {
    // Solution cost.
    logger.info("Objective: " + solution.objectiveValue() + "miles");
    // Inspect solution.
    logger.info("Route:");
    long routeDistance = 0;
    String route = "";
    long index = routing.start(0);
    while (!routing.isEnd(index)) {
      route += manager.indexToNode(index) + " -> ";
      long previousIndex = index;
      index = solution.value(routing.nextVar(index));
      routeDistance += routing.getArcCostForVehicle(previousIndex, index, 0);
    }
    route += manager.indexToNode(routing.end(0));
    logger.info(route);
    logger.info("Route distance: " + routeDistance + "miles");
  }

  public static void main(String[] args) throws Exception {
    Loader.loadNativeLibraries();
    // Instantiate the data problem.
    final DataModel data = new DataModel();

    // Create Routing Index Manager
    RoutingIndexManager manager =
        new RoutingIndexManager(data.distanceMatrix.length, data.vehicleNumber, data.depot);

    // Create Routing Model.
    RoutingModel routing = new RoutingModel(manager);

    // Create and register a transit callback.
    final int transitCallbackIndex =
        routing.registerTransitCallback((long fromIndex, long toIndex) -> {
          // Convert from routing variable Index to user NodeIndex.
          int fromNode = manager.indexToNode(fromIndex);
          int toNode = manager.indexToNode(toIndex);
          return data.distanceMatrix[fromNode][toNode];
        });

    // Define cost of each arc.
    routing.setArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

    // Setting first solution heuristic.
    RoutingSearchParameters searchParameters =
        main.defaultRoutingSearchParameters()
            .toBuilder()
            .setFirstSolutionStrategy(FirstSolutionStrategy.Value.PATH_CHEAPEST_ARC)
            .build();

    // Solve the problem.
    Assignment solution = routing.solveWithParameters(searchParameters);

    // Print solution on console.
    printSolution(routing, manager, solution);
  }
}

C#‎

using System;
using System.Collections.Generic;
using Google.OrTools.ConstraintSolver;

/// <summary>
///   Minimal TSP using distance matrix.
/// </summary>
public class TspCities
{
    class DataModel
    {
        public long[,] DistanceMatrix = {
            { 0, 2451, 713, 1018, 1631, 1374, 2408, 213, 2571, 875, 1420, 2145, 1972 },
            { 2451, 0, 1745, 1524, 831, 1240, 959, 2596, 403, 1589, 1374, 357, 579 },
            { 713, 1745, 0, 355, 920, 803, 1737, 851, 1858, 262, 940, 1453, 1260 },
            { 1018, 1524, 355, 0, 700, 862, 1395, 1123, 1584, 466, 1056, 1280, 987 },
            { 1631, 831, 920, 700, 0, 663, 1021, 1769, 949, 796, 879, 586, 371 },
            { 1374, 1240, 803, 862, 663, 0, 1681, 1551, 1765, 547, 225, 887, 999 },
            { 2408, 959, 1737, 1395, 1021, 1681, 0, 2493, 678, 1724, 1891, 1114, 701 },
            { 213, 2596, 851, 1123, 1769, 1551, 2493, 0, 2699, 1038, 1605, 2300, 2099 },
            { 2571, 403, 1858, 1584, 949, 1765, 678, 2699, 0, 1744, 1645, 653, 600 },
            { 875, 1589, 262, 466, 796, 547, 1724, 1038, 1744, 0, 679, 1272, 1162 },
            { 1420, 1374, 940, 1056, 879, 225, 1891, 1605, 1645, 679, 0, 1017, 1200 },
            { 2145, 357, 1453, 1280, 586, 887, 1114, 2300, 653, 1272, 1017, 0, 504 },
            { 1972, 579, 1260, 987, 371, 999, 701, 2099, 600, 1162, 1200, 504, 0 },
        };
        public int VehicleNumber = 1;
        public int Depot = 0;
    };

    /// <summary>
    ///   Print the solution.
    /// </summary>
    static void PrintSolution(in RoutingModel routing, in RoutingIndexManager manager, in Assignment solution)
    {
        Console.WriteLine("Objective: {0} miles", solution.ObjectiveValue());
        // Inspect solution.
        Console.WriteLine("Route:");
        long routeDistance = 0;
        var index = routing.Start(0);
        while (routing.IsEnd(index) == false)
        {
            Console.Write("{0} -> ", manager.IndexToNode((int)index));
            var previousIndex = index;
            index = solution.Value(routing.NextVar(index));
            routeDistance += routing.GetArcCostForVehicle(previousIndex, index, 0);
        }
        Console.WriteLine("{0}", manager.IndexToNode((int)index));
        Console.WriteLine("Route distance: {0}miles", routeDistance);
    }

    public static void Main(String[] args)
    {
        // Instantiate the data problem.
        DataModel data = new DataModel();

        // Create Routing Index Manager
        RoutingIndexManager manager =
            new RoutingIndexManager(data.DistanceMatrix.GetLength(0), data.VehicleNumber, data.Depot);

        // Create Routing Model.
        RoutingModel routing = new RoutingModel(manager);

        int transitCallbackIndex = routing.RegisterTransitCallback((long fromIndex, long toIndex) =>
                                                                   {
                                                                       // Convert from routing variable Index to
                                                                       // distance matrix NodeIndex.
                                                                       var fromNode = manager.IndexToNode(fromIndex);
                                                                       var toNode = manager.IndexToNode(toIndex);
                                                                       return data.DistanceMatrix[fromNode, toNode];
                                                                   });

        // Define cost of each arc.
        routing.SetArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

        // Setting first solution heuristic.
        RoutingSearchParameters searchParameters =
            operations_research_constraint_solver.DefaultRoutingSearchParameters();
        searchParameters.FirstSolutionStrategy = FirstSolutionStrategy.Types.Value.PathCheapestArc;

        // Solve the problem.
        Assignment solution = routing.SolveWithParameters(searchParameters);

        // Print solution on console.
        PrintSolution(routing, manager, solution);
    }
}

דוגמה: קידוח לוח חשמלי

הדוגמה הבאה כוללת קידוח חורים בלוח מעגל באמצעות מקדח אוטומטי. הבעיה היא למצוא את המסלול הקצר ביותר לקידוח בלוח, כדי לקדוח את החורים הנדרשים. הדוגמה היא מ-TSPLIB – ספרייה של בעיות ב-TSP.

הנה תרשים פיזור עם מיקומי החורים:

בקטעים הבאים מוצגות תוכניות שמוצאות פתרון טוב לבעיה בלוח המעגלים, באמצעות פרמטרי החיפוש שמוגדרים כברירת מחדל בפותר הבעיות. לאחר מכן נראה איך למצוא פתרון טוב יותר על ידי שינוי אסטרטגיית החיפוש.

יצירת הנתונים

נתוני הבעיה כוללים 280 נקודות במטוס, שמוצגות בתרשים הפיזור למעלה. התוכנית יוצרת את הנתונים במגוון צמדים מסודרים, בהתאם לנקודות שבמטוס, כפי שמוצג בהמשך.

Python

def create_data_model():
    """Stores the data for the problem."""
    data = {}
    # Locations in block units
    data["locations"] = [
        # fmt: off
      (288, 149), (288, 129), (270, 133), (256, 141), (256, 157), (246, 157),
      (236, 169), (228, 169), (228, 161), (220, 169), (212, 169), (204, 169),
      (196, 169), (188, 169), (196, 161), (188, 145), (172, 145), (164, 145),
      (156, 145), (148, 145), (140, 145), (148, 169), (164, 169), (172, 169),
      (156, 169), (140, 169), (132, 169), (124, 169), (116, 161), (104, 153),
      (104, 161), (104, 169), (90, 165), (80, 157), (64, 157), (64, 165),
      (56, 169), (56, 161), (56, 153), (56, 145), (56, 137), (56, 129),
      (56, 121), (40, 121), (40, 129), (40, 137), (40, 145), (40, 153),
      (40, 161), (40, 169), (32, 169), (32, 161), (32, 153), (32, 145),
      (32, 137), (32, 129), (32, 121), (32, 113), (40, 113), (56, 113),
      (56, 105), (48, 99), (40, 99), (32, 97), (32, 89), (24, 89),
      (16, 97), (16, 109), (8, 109), (8, 97), (8, 89), (8, 81),
      (8, 73), (8, 65), (8, 57), (16, 57), (8, 49), (8, 41),
      (24, 45), (32, 41), (32, 49), (32, 57), (32, 65), (32, 73),
      (32, 81), (40, 83), (40, 73), (40, 63), (40, 51), (44, 43),
      (44, 35), (44, 27), (32, 25), (24, 25), (16, 25), (16, 17),
      (24, 17), (32, 17), (44, 11), (56, 9), (56, 17), (56, 25),
      (56, 33), (56, 41), (64, 41), (72, 41), (72, 49), (56, 49),
      (48, 51), (56, 57), (56, 65), (48, 63), (48, 73), (56, 73),
      (56, 81), (48, 83), (56, 89), (56, 97), (104, 97), (104, 105),
      (104, 113), (104, 121), (104, 129), (104, 137), (104, 145), (116, 145),
      (124, 145), (132, 145), (132, 137), (140, 137), (148, 137), (156, 137),
      (164, 137), (172, 125), (172, 117), (172, 109), (172, 101), (172, 93),
      (172, 85), (180, 85), (180, 77), (180, 69), (180, 61), (180, 53),
      (172, 53), (172, 61), (172, 69), (172, 77), (164, 81), (148, 85),
      (124, 85), (124, 93), (124, 109), (124, 125), (124, 117), (124, 101),
      (104, 89), (104, 81), (104, 73), (104, 65), (104, 49), (104, 41),
      (104, 33), (104, 25), (104, 17), (92, 9), (80, 9), (72, 9),
      (64, 21), (72, 25), (80, 25), (80, 25), (80, 41), (88, 49),
      (104, 57), (124, 69), (124, 77), (132, 81), (140, 65), (132, 61),
      (124, 61), (124, 53), (124, 45), (124, 37), (124, 29), (132, 21),
      (124, 21), (120, 9), (128, 9), (136, 9), (148, 9), (162, 9),
      (156, 25), (172, 21), (180, 21), (180, 29), (172, 29), (172, 37),
      (172, 45), (180, 45), (180, 37), (188, 41), (196, 49), (204, 57),
      (212, 65), (220, 73), (228, 69), (228, 77), (236, 77), (236, 69),
      (236, 61), (228, 61), (228, 53), (236, 53), (236, 45), (228, 45),
      (228, 37), (236, 37), (236, 29), (228, 29), (228, 21), (236, 21),
      (252, 21), (260, 29), (260, 37), (260, 45), (260, 53), (260, 61),
      (260, 69), (260, 77), (276, 77), (276, 69), (276, 61), (276, 53),
      (284, 53), (284, 61), (284, 69), (284, 77), (284, 85), (284, 93),
      (284, 101), (288, 109), (280, 109), (276, 101), (276, 93), (276, 85),
      (268, 97), (260, 109), (252, 101), (260, 93), (260, 85), (236, 85),
      (228, 85), (228, 93), (236, 93), (236, 101), (228, 101), (228, 109),
      (228, 117), (228, 125), (220, 125), (212, 117), (204, 109), (196, 101),
      (188, 93), (180, 93), (180, 101), (180, 109), (180, 117), (180, 125),
      (196, 145), (204, 145), (212, 145), (220, 145), (228, 145), (236, 145),
      (246, 141), (252, 125), (260, 129), (280, 133)
        # fmt: on
    ]
    data["num_vehicles"] = 1
    data["depot"] = 0
    return data

C++‎

struct DataModel {
  const std::vector<std::vector<int>> locations{
      {288, 149}, {288, 129}, {270, 133}, {256, 141}, {256, 157}, {246, 157},
      {236, 169}, {228, 169}, {228, 161}, {220, 169}, {212, 169}, {204, 169},
      {196, 169}, {188, 169}, {196, 161}, {188, 145}, {172, 145}, {164, 145},
      {156, 145}, {148, 145}, {140, 145}, {148, 169}, {164, 169}, {172, 169},
      {156, 169}, {140, 169}, {132, 169}, {124, 169}, {116, 161}, {104, 153},
      {104, 161}, {104, 169}, {90, 165},  {80, 157},  {64, 157},  {64, 165},
      {56, 169},  {56, 161},  {56, 153},  {56, 145},  {56, 137},  {56, 129},
      {56, 121},  {40, 121},  {40, 129},  {40, 137},  {40, 145},  {40, 153},
      {40, 161},  {40, 169},  {32, 169},  {32, 161},  {32, 153},  {32, 145},
      {32, 137},  {32, 129},  {32, 121},  {32, 113},  {40, 113},  {56, 113},
      {56, 105},  {48, 99},   {40, 99},   {32, 97},   {32, 89},   {24, 89},
      {16, 97},   {16, 109},  {8, 109},   {8, 97},    {8, 89},    {8, 81},
      {8, 73},    {8, 65},    {8, 57},    {16, 57},   {8, 49},    {8, 41},
      {24, 45},   {32, 41},   {32, 49},   {32, 57},   {32, 65},   {32, 73},
      {32, 81},   {40, 83},   {40, 73},   {40, 63},   {40, 51},   {44, 43},
      {44, 35},   {44, 27},   {32, 25},   {24, 25},   {16, 25},   {16, 17},
      {24, 17},   {32, 17},   {44, 11},   {56, 9},    {56, 17},   {56, 25},
      {56, 33},   {56, 41},   {64, 41},   {72, 41},   {72, 49},   {56, 49},
      {48, 51},   {56, 57},   {56, 65},   {48, 63},   {48, 73},   {56, 73},
      {56, 81},   {48, 83},   {56, 89},   {56, 97},   {104, 97},  {104, 105},
      {104, 113}, {104, 121}, {104, 129}, {104, 137}, {104, 145}, {116, 145},
      {124, 145}, {132, 145}, {132, 137}, {140, 137}, {148, 137}, {156, 137},
      {164, 137}, {172, 125}, {172, 117}, {172, 109}, {172, 101}, {172, 93},
      {172, 85},  {180, 85},  {180, 77},  {180, 69},  {180, 61},  {180, 53},
      {172, 53},  {172, 61},  {172, 69},  {172, 77},  {164, 81},  {148, 85},
      {124, 85},  {124, 93},  {124, 109}, {124, 125}, {124, 117}, {124, 101},
      {104, 89},  {104, 81},  {104, 73},  {104, 65},  {104, 49},  {104, 41},
      {104, 33},  {104, 25},  {104, 17},  {92, 9},    {80, 9},    {72, 9},
      {64, 21},   {72, 25},   {80, 25},   {80, 25},   {80, 41},   {88, 49},
      {104, 57},  {124, 69},  {124, 77},  {132, 81},  {140, 65},  {132, 61},
      {124, 61},  {124, 53},  {124, 45},  {124, 37},  {124, 29},  {132, 21},
      {124, 21},  {120, 9},   {128, 9},   {136, 9},   {148, 9},   {162, 9},
      {156, 25},  {172, 21},  {180, 21},  {180, 29},  {172, 29},  {172, 37},
      {172, 45},  {180, 45},  {180, 37},  {188, 41},  {196, 49},  {204, 57},
      {212, 65},  {220, 73},  {228, 69},  {228, 77},  {236, 77},  {236, 69},
      {236, 61},  {228, 61},  {228, 53},  {236, 53},  {236, 45},  {228, 45},
      {228, 37},  {236, 37},  {236, 29},  {228, 29},  {228, 21},  {236, 21},
      {252, 21},  {260, 29},  {260, 37},  {260, 45},  {260, 53},  {260, 61},
      {260, 69},  {260, 77},  {276, 77},  {276, 69},  {276, 61},  {276, 53},
      {284, 53},  {284, 61},  {284, 69},  {284, 77},  {284, 85},  {284, 93},
      {284, 101}, {288, 109}, {280, 109}, {276, 101}, {276, 93},  {276, 85},
      {268, 97},  {260, 109}, {252, 101}, {260, 93},  {260, 85},  {236, 85},
      {228, 85},  {228, 93},  {236, 93},  {236, 101}, {228, 101}, {228, 109},
      {228, 117}, {228, 125}, {220, 125}, {212, 117}, {204, 109}, {196, 101},
      {188, 93},  {180, 93},  {180, 101}, {180, 109}, {180, 117}, {180, 125},
      {196, 145}, {204, 145}, {212, 145}, {220, 145}, {228, 145}, {236, 145},
      {246, 141}, {252, 125}, {260, 129}, {280, 133},
  };
  const int num_vehicles = 1;
  const RoutingIndexManager::NodeIndex depot{0};
};

Java

static class DataModel {
  public final int[][] locations = {{288, 149}, {288, 129}, {270, 133}, {256, 141}, {256, 157},
      {246, 157}, {236, 169}, {228, 169}, {228, 161}, {220, 169}, {212, 169}, {204, 169},
      {196, 169}, {188, 169}, {196, 161}, {188, 145}, {172, 145}, {164, 145}, {156, 145},
      {148, 145}, {140, 145}, {148, 169}, {164, 169}, {172, 169}, {156, 169}, {140, 169},
      {132, 169}, {124, 169}, {116, 161}, {104, 153}, {104, 161}, {104, 169}, {90, 165},
      {80, 157}, {64, 157}, {64, 165}, {56, 169}, {56, 161}, {56, 153}, {56, 145}, {56, 137},
      {56, 129}, {56, 121}, {40, 121}, {40, 129}, {40, 137}, {40, 145}, {40, 153}, {40, 161},
      {40, 169}, {32, 169}, {32, 161}, {32, 153}, {32, 145}, {32, 137}, {32, 129}, {32, 121},
      {32, 113}, {40, 113}, {56, 113}, {56, 105}, {48, 99}, {40, 99}, {32, 97}, {32, 89},
      {24, 89}, {16, 97}, {16, 109}, {8, 109}, {8, 97}, {8, 89}, {8, 81}, {8, 73}, {8, 65},
      {8, 57}, {16, 57}, {8, 49}, {8, 41}, {24, 45}, {32, 41}, {32, 49}, {32, 57}, {32, 65},
      {32, 73}, {32, 81}, {40, 83}, {40, 73}, {40, 63}, {40, 51}, {44, 43}, {44, 35}, {44, 27},
      {32, 25}, {24, 25}, {16, 25}, {16, 17}, {24, 17}, {32, 17}, {44, 11}, {56, 9}, {56, 17},
      {56, 25}, {56, 33}, {56, 41}, {64, 41}, {72, 41}, {72, 49}, {56, 49}, {48, 51}, {56, 57},
      {56, 65}, {48, 63}, {48, 73}, {56, 73}, {56, 81}, {48, 83}, {56, 89}, {56, 97}, {104, 97},
      {104, 105}, {104, 113}, {104, 121}, {104, 129}, {104, 137}, {104, 145}, {116, 145},
      {124, 145}, {132, 145}, {132, 137}, {140, 137}, {148, 137}, {156, 137}, {164, 137},
      {172, 125}, {172, 117}, {172, 109}, {172, 101}, {172, 93}, {172, 85}, {180, 85}, {180, 77},
      {180, 69}, {180, 61}, {180, 53}, {172, 53}, {172, 61}, {172, 69}, {172, 77}, {164, 81},
      {148, 85}, {124, 85}, {124, 93}, {124, 109}, {124, 125}, {124, 117}, {124, 101}, {104, 89},
      {104, 81}, {104, 73}, {104, 65}, {104, 49}, {104, 41}, {104, 33}, {104, 25}, {104, 17},
      {92, 9}, {80, 9}, {72, 9}, {64, 21}, {72, 25}, {80, 25}, {80, 25}, {80, 41}, {88, 49},
      {104, 57}, {124, 69}, {124, 77}, {132, 81}, {140, 65}, {132, 61}, {124, 61}, {124, 53},
      {124, 45}, {124, 37}, {124, 29}, {132, 21}, {124, 21}, {120, 9}, {128, 9}, {136, 9},
      {148, 9}, {162, 9}, {156, 25}, {172, 21}, {180, 21}, {180, 29}, {172, 29}, {172, 37},
      {172, 45}, {180, 45}, {180, 37}, {188, 41}, {196, 49}, {204, 57}, {212, 65}, {220, 73},
      {228, 69}, {228, 77}, {236, 77}, {236, 69}, {236, 61}, {228, 61}, {228, 53}, {236, 53},
      {236, 45}, {228, 45}, {228, 37}, {236, 37}, {236, 29}, {228, 29}, {228, 21}, {236, 21},
      {252, 21}, {260, 29}, {260, 37}, {260, 45}, {260, 53}, {260, 61}, {260, 69}, {260, 77},
      {276, 77}, {276, 69}, {276, 61}, {276, 53}, {284, 53}, {284, 61}, {284, 69}, {284, 77},
      {284, 85}, {284, 93}, {284, 101}, {288, 109}, {280, 109}, {276, 101}, {276, 93}, {276, 85},
      {268, 97}, {260, 109}, {252, 101}, {260, 93}, {260, 85}, {236, 85}, {228, 85}, {228, 93},
      {236, 93}, {236, 101}, {228, 101}, {228, 109}, {228, 117}, {228, 125}, {220, 125},
      {212, 117}, {204, 109}, {196, 101}, {188, 93}, {180, 93}, {180, 101}, {180, 109},
      {180, 117}, {180, 125}, {196, 145}, {204, 145}, {212, 145}, {220, 145}, {228, 145},
      {236, 145}, {246, 141}, {252, 125}, {260, 129}, {280, 133}};
  public final int vehicleNumber = 1;
  public final int depot = 0;
}

C#‎

class DataModel
{
    public int[,] Locations = {
        { 288, 149 }, { 288, 129 }, { 270, 133 }, { 256, 141 }, { 256, 157 }, { 246, 157 }, { 236, 169 },
        { 228, 169 }, { 228, 161 }, { 220, 169 }, { 212, 169 }, { 204, 169 }, { 196, 169 }, { 188, 169 },
        { 196, 161 }, { 188, 145 }, { 172, 145 }, { 164, 145 }, { 156, 145 }, { 148, 145 }, { 140, 145 },
        { 148, 169 }, { 164, 169 }, { 172, 169 }, { 156, 169 }, { 140, 169 }, { 132, 169 }, { 124, 169 },
        { 116, 161 }, { 104, 153 }, { 104, 161 }, { 104, 169 }, { 90, 165 },  { 80, 157 },  { 64, 157 },
        { 64, 165 },  { 56, 169 },  { 56, 161 },  { 56, 153 },  { 56, 145 },  { 56, 137 },  { 56, 129 },
        { 56, 121 },  { 40, 121 },  { 40, 129 },  { 40, 137 },  { 40, 145 },  { 40, 153 },  { 40, 161 },
        { 40, 169 },  { 32, 169 },  { 32, 161 },  { 32, 153 },  { 32, 145 },  { 32, 137 },  { 32, 129 },
        { 32, 121 },  { 32, 113 },  { 40, 113 },  { 56, 113 },  { 56, 105 },  { 48, 99 },   { 40, 99 },
        { 32, 97 },   { 32, 89 },   { 24, 89 },   { 16, 97 },   { 16, 109 },  { 8, 109 },   { 8, 97 },
        { 8, 89 },    { 8, 81 },    { 8, 73 },    { 8, 65 },    { 8, 57 },    { 16, 57 },   { 8, 49 },
        { 8, 41 },    { 24, 45 },   { 32, 41 },   { 32, 49 },   { 32, 57 },   { 32, 65 },   { 32, 73 },
        { 32, 81 },   { 40, 83 },   { 40, 73 },   { 40, 63 },   { 40, 51 },   { 44, 43 },   { 44, 35 },
        { 44, 27 },   { 32, 25 },   { 24, 25 },   { 16, 25 },   { 16, 17 },   { 24, 17 },   { 32, 17 },
        { 44, 11 },   { 56, 9 },    { 56, 17 },   { 56, 25 },   { 56, 33 },   { 56, 41 },   { 64, 41 },
        { 72, 41 },   { 72, 49 },   { 56, 49 },   { 48, 51 },   { 56, 57 },   { 56, 65 },   { 48, 63 },
        { 48, 73 },   { 56, 73 },   { 56, 81 },   { 48, 83 },   { 56, 89 },   { 56, 97 },   { 104, 97 },
        { 104, 105 }, { 104, 113 }, { 104, 121 }, { 104, 129 }, { 104, 137 }, { 104, 145 }, { 116, 145 },
        { 124, 145 }, { 132, 145 }, { 132, 137 }, { 140, 137 }, { 148, 137 }, { 156, 137 }, { 164, 137 },
        { 172, 125 }, { 172, 117 }, { 172, 109 }, { 172, 101 }, { 172, 93 },  { 172, 85 },  { 180, 85 },
        { 180, 77 },  { 180, 69 },  { 180, 61 },  { 180, 53 },  { 172, 53 },  { 172, 61 },  { 172, 69 },
        { 172, 77 },  { 164, 81 },  { 148, 85 },  { 124, 85 },  { 124, 93 },  { 124, 109 }, { 124, 125 },
        { 124, 117 }, { 124, 101 }, { 104, 89 },  { 104, 81 },  { 104, 73 },  { 104, 65 },  { 104, 49 },
        { 104, 41 },  { 104, 33 },  { 104, 25 },  { 104, 17 },  { 92, 9 },    { 80, 9 },    { 72, 9 },
        { 64, 21 },   { 72, 25 },   { 80, 25 },   { 80, 25 },   { 80, 41 },   { 88, 49 },   { 104, 57 },
        { 124, 69 },  { 124, 77 },  { 132, 81 },  { 140, 65 },  { 132, 61 },  { 124, 61 },  { 124, 53 },
        { 124, 45 },  { 124, 37 },  { 124, 29 },  { 132, 21 },  { 124, 21 },  { 120, 9 },   { 128, 9 },
        { 136, 9 },   { 148, 9 },   { 162, 9 },   { 156, 25 },  { 172, 21 },  { 180, 21 },  { 180, 29 },
        { 172, 29 },  { 172, 37 },  { 172, 45 },  { 180, 45 },  { 180, 37 },  { 188, 41 },  { 196, 49 },
        { 204, 57 },  { 212, 65 },  { 220, 73 },  { 228, 69 },  { 228, 77 },  { 236, 77 },  { 236, 69 },
        { 236, 61 },  { 228, 61 },  { 228, 53 },  { 236, 53 },  { 236, 45 },  { 228, 45 },  { 228, 37 },
        { 236, 37 },  { 236, 29 },  { 228, 29 },  { 228, 21 },  { 236, 21 },  { 252, 21 },  { 260, 29 },
        { 260, 37 },  { 260, 45 },  { 260, 53 },  { 260, 61 },  { 260, 69 },  { 260, 77 },  { 276, 77 },
        { 276, 69 },  { 276, 61 },  { 276, 53 },  { 284, 53 },  { 284, 61 },  { 284, 69 },  { 284, 77 },
        { 284, 85 },  { 284, 93 },  { 284, 101 }, { 288, 109 }, { 280, 109 }, { 276, 101 }, { 276, 93 },
        { 276, 85 },  { 268, 97 },  { 260, 109 }, { 252, 101 }, { 260, 93 },  { 260, 85 },  { 236, 85 },
        { 228, 85 },  { 228, 93 },  { 236, 93 },  { 236, 101 }, { 228, 101 }, { 228, 109 }, { 228, 117 },
        { 228, 125 }, { 220, 125 }, { 212, 117 }, { 204, 109 }, { 196, 101 }, { 188, 93 },  { 180, 93 },
        { 180, 101 }, { 180, 109 }, { 180, 117 }, { 180, 125 }, { 196, 145 }, { 204, 145 }, { 212, 145 },
        { 220, 145 }, { 228, 145 }, { 236, 145 }, { 246, 141 }, { 252, 125 }, { 260, 129 }, { 280, 133 },
    };
    public int VehicleNumber = 1;
    public int Depot = 0;
};

חישוב מטריצת המרחק

הפונקציה הבאה מחשבת את המרחק בין האוקלידיים בין שתי נקודות בנתונים, ומאחסנת אותו במערך. מכיוון שפותר הניתוב פועל על המספרים השלמים, הפונקציה מעגלת את המרחקים המחושבים למספרים שלמים. עיגול המספרים לא משפיע על הפתרון בדוגמה הזו, אבל עשוי להופיע במקרים אחרים. כדאי לעיין בשינוי מטריצת המרחק כדי להימנע מבעיות עיגול.

Python

def compute_euclidean_distance_matrix(locations):
    """Creates callback to return distance between points."""
    distances = {}
    for from_counter, from_node in enumerate(locations):
        distances[from_counter] = {}
        for to_counter, to_node in enumerate(locations):
            if from_counter == to_counter:
                distances[from_counter][to_counter] = 0
            else:
                # Euclidean distance
                distances[from_counter][to_counter] = int(
                    math.hypot((from_node[0] - to_node[0]), (from_node[1] - to_node[1]))
                )
    return distances

C++‎

// @brief Generate distance matrix.
std::vector<std::vector<int64_t>> ComputeEuclideanDistanceMatrix(
    const std::vector<std::vector<int>>& locations) {
  std::vector<std::vector<int64_t>> distances =
      std::vector<std::vector<int64_t>>(
          locations.size(), std::vector<int64_t>(locations.size(), int64_t{0}));
  for (int from_node = 0; from_node < locations.size(); from_node++) {
    for (int to_node = 0; to_node < locations.size(); to_node++) {
      if (from_node != to_node)
        distances[from_node][to_node] = static_cast<int64_t>(
            std::hypot((locations[to_node][0] - locations[from_node][0]),
                       (locations[to_node][1] - locations[from_node][1])));
    }
  }
  return distances;
}

Java

/// @brief Compute Euclidean distance matrix from locations array.
/// @details It uses an array of locations and computes
/// the Euclidean distance between any two locations.
private static long[][] computeEuclideanDistanceMatrix(int[][] locations) {
  // Calculate distance matrix using Euclidean distance.
  long[][] distanceMatrix = new long[locations.length][locations.length];
  for (int fromNode = 0; fromNode < locations.length; ++fromNode) {
    for (int toNode = 0; toNode < locations.length; ++toNode) {
      if (fromNode == toNode) {
        distanceMatrix[fromNode][toNode] = 0;
      } else {
        distanceMatrix[fromNode][toNode] =
            (long) Math.hypot(locations[toNode][0] - locations[fromNode][0],
                locations[toNode][1] - locations[fromNode][1]);
      }
    }
  }
  return distanceMatrix;
}

C#‎

/// <summary>
///   Euclidean distance implemented as a callback. It uses an array of
///   positions and computes the Euclidean distance between the two
///   positions of two different indices.
/// </summary>
static long[,] ComputeEuclideanDistanceMatrix(in int[,] locations)
{
    // Calculate the distance matrix using Euclidean distance.
    int locationNumber = locations.GetLength(0);
    long[,] distanceMatrix = new long[locationNumber, locationNumber];
    for (int fromNode = 0; fromNode < locationNumber; fromNode++)
    {
        for (int toNode = 0; toNode < locationNumber; toNode++)
        {
            if (fromNode == toNode)
                distanceMatrix[fromNode, toNode] = 0;
            else
                distanceMatrix[fromNode, toNode] =
                    (long)Math.Sqrt(Math.Pow(locations[toNode, 0] - locations[fromNode, 0], 2) +
                                    Math.Pow(locations[toNode, 1] - locations[fromNode, 1], 2));
        }
    }
    return distanceMatrix;
}

הוספת הקריאה החוזרת (callback) של המרחק

הקוד שיוצר את הקריאה החוזרת (callback) מרחוק כמעט זהה לקוד שבדוגמה הקודמת. עם זאת, במקרה כזה התוכנית קוראת לפונקציה שמחשבון את מטריצת המרחק לפני הוספת הקריאה החוזרת.

Python

distance_matrix = compute_euclidean_distance_matrix(data["locations"])

def distance_callback(from_index, to_index):
    """Returns the distance between the two nodes."""
    # Convert from routing variable Index to distance matrix NodeIndex.
    from_node = manager.IndexToNode(from_index)
    to_node = manager.IndexToNode(to_index)
    return distance_matrix[from_node][to_node]

transit_callback_index = routing.RegisterTransitCallback(distance_callback)
routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index)

C++‎

const auto distance_matrix = ComputeEuclideanDistanceMatrix(data.locations);
const int transit_callback_index = routing.RegisterTransitCallback(
    [&distance_matrix, &manager](const int64_t from_index,
                                 const int64_t to_index) -> int64_t {
      // Convert from routing variable Index to distance matrix NodeIndex.
      const int from_node = manager.IndexToNode(from_index).value();
      const int to_node = manager.IndexToNode(to_index).value();
      return distance_matrix[from_node][to_node];
    });
routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index);

Java

final long[][] distanceMatrix = computeEuclideanDistanceMatrix(data.locations);
final int transitCallbackIndex =
    routing.registerTransitCallback((long fromIndex, long toIndex) -> {
      // Convert from routing variable Index to user NodeIndex.
      int fromNode = manager.indexToNode(fromIndex);
      int toNode = manager.indexToNode(toIndex);
      return distanceMatrix[fromNode][toNode];
    });
routing.setArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

C#‎

long[,] distanceMatrix = ComputeEuclideanDistanceMatrix(data.Locations);
int transitCallbackIndex = routing.RegisterTransitCallback((long fromIndex, long toIndex) =>
                                                           {
                                                               // Convert from routing variable Index to
                                                               // distance matrix NodeIndex.
                                                               var fromNode = manager.IndexToNode(fromIndex);
                                                               var toNode = manager.IndexToNode(toIndex);
                                                               return distanceMatrix[fromNode, toNode];
                                                           });
routing.SetArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

מדפסת פתרונות

הפונקציה הבאה מדפיסה את הפתרון למסוף. כדי שהפלט יהיה קומפקטי יותר, הפונקציה מציגה רק את האינדקסים של המיקומים במסלול.

Python

def print_solution(manager, routing, solution):
    """Prints solution on console."""
    print(f"Objective: {solution.ObjectiveValue()}")
    index = routing.Start(0)
    plan_output = "Route:\n"
    route_distance = 0
    while not routing.IsEnd(index):
        plan_output += f" {manager.IndexToNode(index)} ->"
        previous_index = index
        index = solution.Value(routing.NextVar(index))
        route_distance += routing.GetArcCostForVehicle(previous_index, index, 0)
    plan_output += f" {manager.IndexToNode(index)}\n"
    print(plan_output)
    plan_output += f"Objective: {route_distance}m\n"

C++‎

//! @brief Print the solution
//! @param[in] manager Index manager used.
//! @param[in] routing Routing solver used.
//! @param[in] solution Solution found by the solver.
void PrintSolution(const RoutingIndexManager& manager,
                   const RoutingModel& routing, const Assignment& solution) {
  LOG(INFO) << "Objective: " << solution.ObjectiveValue();
  // Inspect solution.
  int64_t index = routing.Start(0);
  LOG(INFO) << "Route:";
  int64_t distance{0};
  std::stringstream route;
  while (!routing.IsEnd(index)) {
    route << manager.IndexToNode(index).value() << " -> ";
    const int64_t previous_index = index;
    index = solution.Value(routing.NextVar(index));
    distance += routing.GetArcCostForVehicle(previous_index, index, int64_t{0});
  }
  LOG(INFO) << route.str() << manager.IndexToNode(index).value();
  LOG(INFO) << "Route distance: " << distance << "miles";
  LOG(INFO) << "";
  LOG(INFO) << "Advanced usage:";
  LOG(INFO) << "Problem solved in " << routing.solver()->wall_time() << "ms";
}

Java

/// @brief Print the solution.
static void printSolution(
    RoutingModel routing, RoutingIndexManager manager, Assignment solution) {
  // Solution cost.
  logger.info("Objective: " + solution.objectiveValue());
  // Inspect solution.
  logger.info("Route:");
  long routeDistance = 0;
  String route = "";
  long index = routing.start(0);
  while (!routing.isEnd(index)) {
    route += manager.indexToNode(index) + " -> ";
    long previousIndex = index;
    index = solution.value(routing.nextVar(index));
    routing.getArcCostForVehicle(previousIndex, index, 0);
  }
  route += manager.indexToNode(routing.end(0));
  logger.info(route);
  logger.info("Route distance: " + routeDistance);
}

C#‎

/// <summary>
///   Print the solution.
/// </summary>
static void PrintSolution(in RoutingModel routing, in RoutingIndexManager manager, in Assignment solution)
{
    Console.WriteLine("Objective: {0}", solution.ObjectiveValue());
    // Inspect solution.
    Console.WriteLine("Route:");
    long routeDistance = 0;
    var index = routing.Start(0);
    while (routing.IsEnd(index) == false)
    {
        Console.Write("{0} -> ", manager.IndexToNode((int)index));
        var previousIndex = index;
        index = solution.Value(routing.NextVar(index));
        routeDistance += routing.GetArcCostForVehicle(previousIndex, index, 0);
    }
    Console.WriteLine("{0}", manager.IndexToNode((int)index));
    Console.WriteLine("Route distance: {0}m", routeDistance);
}

התפקיד הראשי

הפונקציה הראשית זהה למעשה לפונקציה שבדוגמה הקודמת, אבל היא כוללת גם קריאה לפונקציה שיוצרת את מטריצת המרחקים.

הפעלת התוכנית

כל התוכניות מוצגות בקטע הבא. בעת הפעלת התוכנית, מוצג הנתיב הבא:

Total distance: 2790

Route of vehicle 0:
0 -> 1 -> 279 -> 2 -> 278 -> 277 -> 247 -> 248 -> 249 -> 246 -> 244 -> 243 -> 242 -> 241 -> 240 ->
239 -> 238 -> 237 -> 236 -> 235 -> 234 -> 233 -> 232 -> 231 -> 230 -> 245 -> 250 -> 229 -> 228 ->
227 -> 226 -> 225 -> 224 -> 223 -> 222 -> 221 -> 220 -> 219 -> 218 -> 217 -> 216 -> 215 -> 214 ->
213 -> 212 -> 211 -> 210 -> 209 -> 208 -> 251 -> 254 -> 255 -> 257 -> 256 -> 253 -> 252 -> 207 ->
206 -> 205 -> 204 -> 203 -> 202 -> 142 -> 141 -> 146 -> 147 -> 140 -> 139 -> 265 -> 136 -> 137 ->
138 -> 148 -> 149 -> 177 -> 176 -> 175 -> 178 -> 179 -> 180 -> 181 -> 182 -> 183 -> 184 -> 186 ->
185 -> 192 -> 196 -> 197 -> 198 -> 144 -> 145 -> 143 -> 199 -> 201 -> 200 -> 195 -> 194 -> 193 ->
191 -> 190 -> 189 -> 188 -> 187 -> 163 -> 164 -> 165 -> 166 -> 167 -> 168 -> 169 -> 171 -> 170 ->
172 -> 105 -> 106 -> 104 -> 103 -> 107 -> 109 -> 110 -> 113 -> 114 -> 116 -> 117 -> 61 -> 62 ->
63 -> 65 -> 64 -> 84 -> 85 -> 115 -> 112 -> 86 -> 83 -> 82 -> 87 -> 111 -> 108 -> 89 -> 90 -> 91 ->
102 -> 101 -> 100 -> 99 -> 98 -> 97 -> 96 -> 95 -> 94 -> 93 -> 92 -> 79 -> 88 -> 81 -> 80 -> 78 ->
77 -> 76 -> 74 -> 75 -> 73 -> 72 -> 71 -> 70 -> 69 -> 66 -> 68 -> 67 -> 57 -> 56 -> 55 -> 54 ->
53 -> 52 -> 51 -> 50 -> 49 -> 48 -> 47 -> 46 -> 45 -> 44 -> 43 -> 58 -> 60 -> 59 -> 42 -> 41 ->
40 -> 39 -> 38 -> 37 -> 36 -> 35 -> 34 -> 33 -> 32 -> 31 -> 30 -> 29 -> 124 -> 123 -> 122 -> 121 ->
120 -> 119 -> 118 -> 156 -> 157 -> 158 -> 173 -> 162 -> 161 -> 160 -> 174 -> 159 -> 150 -> 151 ->
155 -> 152 -> 154 -> 153 -> 128 -> 129 -> 130 -> 131 -> 18 -> 19 -> 20 -> 127 -> 126 -> 125 -> 28 ->
27 -> 26 -> 25 -> 21 -> 24 -> 22 -> 23 -> 13 -> 12 -> 14 -> 11 -> 10 -> 9 -> 7 -> 8 -> 6 -> 5 ->
275 -> 274 -> 273 -> 272 -> 271 -> 270 -> 15 -> 16 -> 17 -> 132 -> 133 -> 269 -> 268 -> 134 ->
135 -> 267 -> 266 -> 264 -> 263 -> 262 -> 261 -> 260 -> 258 -> 259 -> 276 -> 3 -> 4 -> 0

הנה תרשים של המסלול התואם:

ספריית OR-Tools מוצאת את הסיור שלמעלה במהירות רבה: תוך פחות משנייה במחשב רגיל. האורך הכולל של הסיור שלמעלה הוא 2,790.

השלמת התוכניות

כאן מוצגות התוכניות המלאות לדוגמה של הלוח.

Python

"""Simple Travelling Salesperson Problem (TSP) on a circuit board."""

import math
from ortools.constraint_solver import routing_enums_pb2
from ortools.constraint_solver import pywrapcp


def create_data_model():
    """Stores the data for the problem."""
    data = {}
    # Locations in block units
    data["locations"] = [
        # fmt: off
      (288, 149), (288, 129), (270, 133), (256, 141), (256, 157), (246, 157),
      (236, 169), (228, 169), (228, 161), (220, 169), (212, 169), (204, 169),
      (196, 169), (188, 169), (196, 161), (188, 145), (172, 145), (164, 145),
      (156, 145), (148, 145), (140, 145), (148, 169), (164, 169), (172, 169),
      (156, 169), (140, 169), (132, 169), (124, 169), (116, 161), (104, 153),
      (104, 161), (104, 169), (90, 165), (80, 157), (64, 157), (64, 165),
      (56, 169), (56, 161), (56, 153), (56, 145), (56, 137), (56, 129),
      (56, 121), (40, 121), (40, 129), (40, 137), (40, 145), (40, 153),
      (40, 161), (40, 169), (32, 169), (32, 161), (32, 153), (32, 145),
      (32, 137), (32, 129), (32, 121), (32, 113), (40, 113), (56, 113),
      (56, 105), (48, 99), (40, 99), (32, 97), (32, 89), (24, 89),
      (16, 97), (16, 109), (8, 109), (8, 97), (8, 89), (8, 81),
      (8, 73), (8, 65), (8, 57), (16, 57), (8, 49), (8, 41),
      (24, 45), (32, 41), (32, 49), (32, 57), (32, 65), (32, 73),
      (32, 81), (40, 83), (40, 73), (40, 63), (40, 51), (44, 43),
      (44, 35), (44, 27), (32, 25), (24, 25), (16, 25), (16, 17),
      (24, 17), (32, 17), (44, 11), (56, 9), (56, 17), (56, 25),
      (56, 33), (56, 41), (64, 41), (72, 41), (72, 49), (56, 49),
      (48, 51), (56, 57), (56, 65), (48, 63), (48, 73), (56, 73),
      (56, 81), (48, 83), (56, 89), (56, 97), (104, 97), (104, 105),
      (104, 113), (104, 121), (104, 129), (104, 137), (104, 145), (116, 145),
      (124, 145), (132, 145), (132, 137), (140, 137), (148, 137), (156, 137),
      (164, 137), (172, 125), (172, 117), (172, 109), (172, 101), (172, 93),
      (172, 85), (180, 85), (180, 77), (180, 69), (180, 61), (180, 53),
      (172, 53), (172, 61), (172, 69), (172, 77), (164, 81), (148, 85),
      (124, 85), (124, 93), (124, 109), (124, 125), (124, 117), (124, 101),
      (104, 89), (104, 81), (104, 73), (104, 65), (104, 49), (104, 41),
      (104, 33), (104, 25), (104, 17), (92, 9), (80, 9), (72, 9),
      (64, 21), (72, 25), (80, 25), (80, 25), (80, 41), (88, 49),
      (104, 57), (124, 69), (124, 77), (132, 81), (140, 65), (132, 61),
      (124, 61), (124, 53), (124, 45), (124, 37), (124, 29), (132, 21),
      (124, 21), (120, 9), (128, 9), (136, 9), (148, 9), (162, 9),
      (156, 25), (172, 21), (180, 21), (180, 29), (172, 29), (172, 37),
      (172, 45), (180, 45), (180, 37), (188, 41), (196, 49), (204, 57),
      (212, 65), (220, 73), (228, 69), (228, 77), (236, 77), (236, 69),
      (236, 61), (228, 61), (228, 53), (236, 53), (236, 45), (228, 45),
      (228, 37), (236, 37), (236, 29), (228, 29), (228, 21), (236, 21),
      (252, 21), (260, 29), (260, 37), (260, 45), (260, 53), (260, 61),
      (260, 69), (260, 77), (276, 77), (276, 69), (276, 61), (276, 53),
      (284, 53), (284, 61), (284, 69), (284, 77), (284, 85), (284, 93),
      (284, 101), (288, 109), (280, 109), (276, 101), (276, 93), (276, 85),
      (268, 97), (260, 109), (252, 101), (260, 93), (260, 85), (236, 85),
      (228, 85), (228, 93), (236, 93), (236, 101), (228, 101), (228, 109),
      (228, 117), (228, 125), (220, 125), (212, 117), (204, 109), (196, 101),
      (188, 93), (180, 93), (180, 101), (180, 109), (180, 117), (180, 125),
      (196, 145), (204, 145), (212, 145), (220, 145), (228, 145), (236, 145),
      (246, 141), (252, 125), (260, 129), (280, 133)
        # fmt: on
    ]
    data["num_vehicles"] = 1
    data["depot"] = 0
    return data


def compute_euclidean_distance_matrix(locations):
    """Creates callback to return distance between points."""
    distances = {}
    for from_counter, from_node in enumerate(locations):
        distances[from_counter] = {}
        for to_counter, to_node in enumerate(locations):
            if from_counter == to_counter:
                distances[from_counter][to_counter] = 0
            else:
                # Euclidean distance
                distances[from_counter][to_counter] = int(
                    math.hypot((from_node[0] - to_node[0]), (from_node[1] - to_node[1]))
                )
    return distances


def print_solution(manager, routing, solution):
    """Prints solution on console."""
    print(f"Objective: {solution.ObjectiveValue()}")
    index = routing.Start(0)
    plan_output = "Route:\n"
    route_distance = 0
    while not routing.IsEnd(index):
        plan_output += f" {manager.IndexToNode(index)} ->"
        previous_index = index
        index = solution.Value(routing.NextVar(index))
        route_distance += routing.GetArcCostForVehicle(previous_index, index, 0)
    plan_output += f" {manager.IndexToNode(index)}\n"
    print(plan_output)
    plan_output += f"Objective: {route_distance}m\n"


def main():
    """Entry point of the program."""
    # Instantiate the data problem.
    data = create_data_model()

    # Create the routing index manager.
    manager = pywrapcp.RoutingIndexManager(
        len(data["locations"]), data["num_vehicles"], data["depot"]
    )

    # Create Routing Model.
    routing = pywrapcp.RoutingModel(manager)

    distance_matrix = compute_euclidean_distance_matrix(data["locations"])

    def distance_callback(from_index, to_index):
        """Returns the distance between the two nodes."""
        # Convert from routing variable Index to distance matrix NodeIndex.
        from_node = manager.IndexToNode(from_index)
        to_node = manager.IndexToNode(to_index)
        return distance_matrix[from_node][to_node]

    transit_callback_index = routing.RegisterTransitCallback(distance_callback)

    # Define cost of each arc.
    routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index)

    # Setting first solution heuristic.
    search_parameters = pywrapcp.DefaultRoutingSearchParameters()
    search_parameters.first_solution_strategy = (
        routing_enums_pb2.FirstSolutionStrategy.PATH_CHEAPEST_ARC
    )

    # Solve the problem.
    solution = routing.SolveWithParameters(search_parameters)

    # Print solution on console.
    if solution:
        print_solution(manager, routing, solution)


if __name__ == "__main__":
    main()

C++‎

#include <cmath>
#include <cstdint>
#include <sstream>
#include <vector>

#include "ortools/constraint_solver/routing.h"
#include "ortools/constraint_solver/routing_enums.pb.h"
#include "ortools/constraint_solver/routing_index_manager.h"
#include "ortools/constraint_solver/routing_parameters.h"

namespace operations_research {
struct DataModel {
  const std::vector<std::vector<int>> locations{
      {288, 149}, {288, 129}, {270, 133}, {256, 141}, {256, 157}, {246, 157},
      {236, 169}, {228, 169}, {228, 161}, {220, 169}, {212, 169}, {204, 169},
      {196, 169}, {188, 169}, {196, 161}, {188, 145}, {172, 145}, {164, 145},
      {156, 145}, {148, 145}, {140, 145}, {148, 169}, {164, 169}, {172, 169},
      {156, 169}, {140, 169}, {132, 169}, {124, 169}, {116, 161}, {104, 153},
      {104, 161}, {104, 169}, {90, 165},  {80, 157},  {64, 157},  {64, 165},
      {56, 169},  {56, 161},  {56, 153},  {56, 145},  {56, 137},  {56, 129},
      {56, 121},  {40, 121},  {40, 129},  {40, 137},  {40, 145},  {40, 153},
      {40, 161},  {40, 169},  {32, 169},  {32, 161},  {32, 153},  {32, 145},
      {32, 137},  {32, 129},  {32, 121},  {32, 113},  {40, 113},  {56, 113},
      {56, 105},  {48, 99},   {40, 99},   {32, 97},   {32, 89},   {24, 89},
      {16, 97},   {16, 109},  {8, 109},   {8, 97},    {8, 89},    {8, 81},
      {8, 73},    {8, 65},    {8, 57},    {16, 57},   {8, 49},    {8, 41},
      {24, 45},   {32, 41},   {32, 49},   {32, 57},   {32, 65},   {32, 73},
      {32, 81},   {40, 83},   {40, 73},   {40, 63},   {40, 51},   {44, 43},
      {44, 35},   {44, 27},   {32, 25},   {24, 25},   {16, 25},   {16, 17},
      {24, 17},   {32, 17},   {44, 11},   {56, 9},    {56, 17},   {56, 25},
      {56, 33},   {56, 41},   {64, 41},   {72, 41},   {72, 49},   {56, 49},
      {48, 51},   {56, 57},   {56, 65},   {48, 63},   {48, 73},   {56, 73},
      {56, 81},   {48, 83},   {56, 89},   {56, 97},   {104, 97},  {104, 105},
      {104, 113}, {104, 121}, {104, 129}, {104, 137}, {104, 145}, {116, 145},
      {124, 145}, {132, 145}, {132, 137}, {140, 137}, {148, 137}, {156, 137},
      {164, 137}, {172, 125}, {172, 117}, {172, 109}, {172, 101}, {172, 93},
      {172, 85},  {180, 85},  {180, 77},  {180, 69},  {180, 61},  {180, 53},
      {172, 53},  {172, 61},  {172, 69},  {172, 77},  {164, 81},  {148, 85},
      {124, 85},  {124, 93},  {124, 109}, {124, 125}, {124, 117}, {124, 101},
      {104, 89},  {104, 81},  {104, 73},  {104, 65},  {104, 49},  {104, 41},
      {104, 33},  {104, 25},  {104, 17},  {92, 9},    {80, 9},    {72, 9},
      {64, 21},   {72, 25},   {80, 25},   {80, 25},   {80, 41},   {88, 49},
      {104, 57},  {124, 69},  {124, 77},  {132, 81},  {140, 65},  {132, 61},
      {124, 61},  {124, 53},  {124, 45},  {124, 37},  {124, 29},  {132, 21},
      {124, 21},  {120, 9},   {128, 9},   {136, 9},   {148, 9},   {162, 9},
      {156, 25},  {172, 21},  {180, 21},  {180, 29},  {172, 29},  {172, 37},
      {172, 45},  {180, 45},  {180, 37},  {188, 41},  {196, 49},  {204, 57},
      {212, 65},  {220, 73},  {228, 69},  {228, 77},  {236, 77},  {236, 69},
      {236, 61},  {228, 61},  {228, 53},  {236, 53},  {236, 45},  {228, 45},
      {228, 37},  {236, 37},  {236, 29},  {228, 29},  {228, 21},  {236, 21},
      {252, 21},  {260, 29},  {260, 37},  {260, 45},  {260, 53},  {260, 61},
      {260, 69},  {260, 77},  {276, 77},  {276, 69},  {276, 61},  {276, 53},
      {284, 53},  {284, 61},  {284, 69},  {284, 77},  {284, 85},  {284, 93},
      {284, 101}, {288, 109}, {280, 109}, {276, 101}, {276, 93},  {276, 85},
      {268, 97},  {260, 109}, {252, 101}, {260, 93},  {260, 85},  {236, 85},
      {228, 85},  {228, 93},  {236, 93},  {236, 101}, {228, 101}, {228, 109},
      {228, 117}, {228, 125}, {220, 125}, {212, 117}, {204, 109}, {196, 101},
      {188, 93},  {180, 93},  {180, 101}, {180, 109}, {180, 117}, {180, 125},
      {196, 145}, {204, 145}, {212, 145}, {220, 145}, {228, 145}, {236, 145},
      {246, 141}, {252, 125}, {260, 129}, {280, 133},
  };
  const int num_vehicles = 1;
  const RoutingIndexManager::NodeIndex depot{0};
};

// @brief Generate distance matrix.
std::vector<std::vector<int64_t>> ComputeEuclideanDistanceMatrix(
    const std::vector<std::vector<int>>& locations) {
  std::vector<std::vector<int64_t>> distances =
      std::vector<std::vector<int64_t>>(
          locations.size(), std::vector<int64_t>(locations.size(), int64_t{0}));
  for (int from_node = 0; from_node < locations.size(); from_node++) {
    for (int to_node = 0; to_node < locations.size(); to_node++) {
      if (from_node != to_node)
        distances[from_node][to_node] = static_cast<int64_t>(
            std::hypot((locations[to_node][0] - locations[from_node][0]),
                       (locations[to_node][1] - locations[from_node][1])));
    }
  }
  return distances;
}

//! @brief Print the solution
//! @param[in] manager Index manager used.
//! @param[in] routing Routing solver used.
//! @param[in] solution Solution found by the solver.
void PrintSolution(const RoutingIndexManager& manager,
                   const RoutingModel& routing, const Assignment& solution) {
  LOG(INFO) << "Objective: " << solution.ObjectiveValue();
  // Inspect solution.
  int64_t index = routing.Start(0);
  LOG(INFO) << "Route:";
  int64_t distance{0};
  std::stringstream route;
  while (!routing.IsEnd(index)) {
    route << manager.IndexToNode(index).value() << " -> ";
    const int64_t previous_index = index;
    index = solution.Value(routing.NextVar(index));
    distance += routing.GetArcCostForVehicle(previous_index, index, int64_t{0});
  }
  LOG(INFO) << route.str() << manager.IndexToNode(index).value();
  LOG(INFO) << "Route distance: " << distance << "miles";
  LOG(INFO) << "";
  LOG(INFO) << "Advanced usage:";
  LOG(INFO) << "Problem solved in " << routing.solver()->wall_time() << "ms";
}

void Tsp() {
  // Instantiate the data problem.
  DataModel data;

  // Create Routing Index Manager
  RoutingIndexManager manager(data.locations.size(), data.num_vehicles,
                              data.depot);

  // Create Routing Model.
  RoutingModel routing(manager);

  const auto distance_matrix = ComputeEuclideanDistanceMatrix(data.locations);
  const int transit_callback_index = routing.RegisterTransitCallback(
      [&distance_matrix, &manager](const int64_t from_index,
                                   const int64_t to_index) -> int64_t {
        // Convert from routing variable Index to distance matrix NodeIndex.
        const int from_node = manager.IndexToNode(from_index).value();
        const int to_node = manager.IndexToNode(to_index).value();
        return distance_matrix[from_node][to_node];
      });

  // Define cost of each arc.
  routing.SetArcCostEvaluatorOfAllVehicles(transit_callback_index);

  // Setting first solution heuristic.
  RoutingSearchParameters searchParameters = DefaultRoutingSearchParameters();
  searchParameters.set_first_solution_strategy(
      FirstSolutionStrategy::PATH_CHEAPEST_ARC);

  // Solve the problem.
  const Assignment* solution = routing.SolveWithParameters(searchParameters);

  // Print solution on console.
  PrintSolution(manager, routing, *solution);
}
}  // namespace operations_research

int main(int /*argc*/, char* /*argv*/[]) {
  operations_research::Tsp();
  return EXIT_SUCCESS;
}

Java

package com.google.ortools.constraintsolver.samples;
import com.google.ortools.Loader;
import com.google.ortools.constraintsolver.Assignment;
import com.google.ortools.constraintsolver.FirstSolutionStrategy;
import com.google.ortools.constraintsolver.RoutingIndexManager;
import com.google.ortools.constraintsolver.RoutingModel;
import com.google.ortools.constraintsolver.RoutingSearchParameters;
import com.google.ortools.constraintsolver.main;
import java.util.logging.Logger;


/** Minimal TSP. */
public class TspCircuitBoard {
  private static final Logger logger = Logger.getLogger(TspCircuitBoard.class.getName());

  static class DataModel {
    public final int[][] locations = {{288, 149}, {288, 129}, {270, 133}, {256, 141}, {256, 157},
        {246, 157}, {236, 169}, {228, 169}, {228, 161}, {220, 169}, {212, 169}, {204, 169},
        {196, 169}, {188, 169}, {196, 161}, {188, 145}, {172, 145}, {164, 145}, {156, 145},
        {148, 145}, {140, 145}, {148, 169}, {164, 169}, {172, 169}, {156, 169}, {140, 169},
        {132, 169}, {124, 169}, {116, 161}, {104, 153}, {104, 161}, {104, 169}, {90, 165},
        {80, 157}, {64, 157}, {64, 165}, {56, 169}, {56, 161}, {56, 153}, {56, 145}, {56, 137},
        {56, 129}, {56, 121}, {40, 121}, {40, 129}, {40, 137}, {40, 145}, {40, 153}, {40, 161},
        {40, 169}, {32, 169}, {32, 161}, {32, 153}, {32, 145}, {32, 137}, {32, 129}, {32, 121},
        {32, 113}, {40, 113}, {56, 113}, {56, 105}, {48, 99}, {40, 99}, {32, 97}, {32, 89},
        {24, 89}, {16, 97}, {16, 109}, {8, 109}, {8, 97}, {8, 89}, {8, 81}, {8, 73}, {8, 65},
        {8, 57}, {16, 57}, {8, 49}, {8, 41}, {24, 45}, {32, 41}, {32, 49}, {32, 57}, {32, 65},
        {32, 73}, {32, 81}, {40, 83}, {40, 73}, {40, 63}, {40, 51}, {44, 43}, {44, 35}, {44, 27},
        {32, 25}, {24, 25}, {16, 25}, {16, 17}, {24, 17}, {32, 17}, {44, 11}, {56, 9}, {56, 17},
        {56, 25}, {56, 33}, {56, 41}, {64, 41}, {72, 41}, {72, 49}, {56, 49}, {48, 51}, {56, 57},
        {56, 65}, {48, 63}, {48, 73}, {56, 73}, {56, 81}, {48, 83}, {56, 89}, {56, 97}, {104, 97},
        {104, 105}, {104, 113}, {104, 121}, {104, 129}, {104, 137}, {104, 145}, {116, 145},
        {124, 145}, {132, 145}, {132, 137}, {140, 137}, {148, 137}, {156, 137}, {164, 137},
        {172, 125}, {172, 117}, {172, 109}, {172, 101}, {172, 93}, {172, 85}, {180, 85}, {180, 77},
        {180, 69}, {180, 61}, {180, 53}, {172, 53}, {172, 61}, {172, 69}, {172, 77}, {164, 81},
        {148, 85}, {124, 85}, {124, 93}, {124, 109}, {124, 125}, {124, 117}, {124, 101}, {104, 89},
        {104, 81}, {104, 73}, {104, 65}, {104, 49}, {104, 41}, {104, 33}, {104, 25}, {104, 17},
        {92, 9}, {80, 9}, {72, 9}, {64, 21}, {72, 25}, {80, 25}, {80, 25}, {80, 41}, {88, 49},
        {104, 57}, {124, 69}, {124, 77}, {132, 81}, {140, 65}, {132, 61}, {124, 61}, {124, 53},
        {124, 45}, {124, 37}, {124, 29}, {132, 21}, {124, 21}, {120, 9}, {128, 9}, {136, 9},
        {148, 9}, {162, 9}, {156, 25}, {172, 21}, {180, 21}, {180, 29}, {172, 29}, {172, 37},
        {172, 45}, {180, 45}, {180, 37}, {188, 41}, {196, 49}, {204, 57}, {212, 65}, {220, 73},
        {228, 69}, {228, 77}, {236, 77}, {236, 69}, {236, 61}, {228, 61}, {228, 53}, {236, 53},
        {236, 45}, {228, 45}, {228, 37}, {236, 37}, {236, 29}, {228, 29}, {228, 21}, {236, 21},
        {252, 21}, {260, 29}, {260, 37}, {260, 45}, {260, 53}, {260, 61}, {260, 69}, {260, 77},
        {276, 77}, {276, 69}, {276, 61}, {276, 53}, {284, 53}, {284, 61}, {284, 69}, {284, 77},
        {284, 85}, {284, 93}, {284, 101}, {288, 109}, {280, 109}, {276, 101}, {276, 93}, {276, 85},
        {268, 97}, {260, 109}, {252, 101}, {260, 93}, {260, 85}, {236, 85}, {228, 85}, {228, 93},
        {236, 93}, {236, 101}, {228, 101}, {228, 109}, {228, 117}, {228, 125}, {220, 125},
        {212, 117}, {204, 109}, {196, 101}, {188, 93}, {180, 93}, {180, 101}, {180, 109},
        {180, 117}, {180, 125}, {196, 145}, {204, 145}, {212, 145}, {220, 145}, {228, 145},
        {236, 145}, {246, 141}, {252, 125}, {260, 129}, {280, 133}};
    public final int vehicleNumber = 1;
    public final int depot = 0;
  }

  /// @brief Compute Euclidean distance matrix from locations array.
  /// @details It uses an array of locations and computes
  /// the Euclidean distance between any two locations.
  private static long[][] computeEuclideanDistanceMatrix(int[][] locations) {
    // Calculate distance matrix using Euclidean distance.
    long[][] distanceMatrix = new long[locations.length][locations.length];
    for (int fromNode = 0; fromNode < locations.length; ++fromNode) {
      for (int toNode = 0; toNode < locations.length; ++toNode) {
        if (fromNode == toNode) {
          distanceMatrix[fromNode][toNode] = 0;
        } else {
          distanceMatrix[fromNode][toNode] =
              (long) Math.hypot(locations[toNode][0] - locations[fromNode][0],
                  locations[toNode][1] - locations[fromNode][1]);
        }
      }
    }
    return distanceMatrix;
  }

  /// @brief Print the solution.
  static void printSolution(
      RoutingModel routing, RoutingIndexManager manager, Assignment solution) {
    // Solution cost.
    logger.info("Objective: " + solution.objectiveValue());
    // Inspect solution.
    logger.info("Route:");
    long routeDistance = 0;
    String route = "";
    long index = routing.start(0);
    while (!routing.isEnd(index)) {
      route += manager.indexToNode(index) + " -> ";
      long previousIndex = index;
      index = solution.value(routing.nextVar(index));
      routing.getArcCostForVehicle(previousIndex, index, 0);
    }
    route += manager.indexToNode(routing.end(0));
    logger.info(route);
    logger.info("Route distance: " + routeDistance);
  }

  public static void main(String[] args) throws Exception {
    Loader.loadNativeLibraries();
    // Instantiate the data problem.
    final DataModel data = new DataModel();

    // Create Routing Index Manager
    RoutingIndexManager manager =
        new RoutingIndexManager(data.locations.length, data.vehicleNumber, data.depot);

    // Create Routing Model.
    RoutingModel routing = new RoutingModel(manager);

    // Create and register a transit callback.
    final long[][] distanceMatrix = computeEuclideanDistanceMatrix(data.locations);
    final int transitCallbackIndex =
        routing.registerTransitCallback((long fromIndex, long toIndex) -> {
          // Convert from routing variable Index to user NodeIndex.
          int fromNode = manager.indexToNode(fromIndex);
          int toNode = manager.indexToNode(toIndex);
          return distanceMatrix[fromNode][toNode];
        });

    // Define cost of each arc.
    routing.setArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

    // Setting first solution heuristic.
    RoutingSearchParameters searchParameters =
        main.defaultRoutingSearchParameters()
            .toBuilder()
            .setFirstSolutionStrategy(FirstSolutionStrategy.Value.PATH_CHEAPEST_ARC)
            .build();

    // Solve the problem.
    Assignment solution = routing.solveWithParameters(searchParameters);

    // Print solution on console.
    printSolution(routing, manager, solution);
  }
}

C#‎

using System;
using System.Collections.Generic;
using Google.OrTools.ConstraintSolver;

/// <summary>
///   Minimal TSP.
///   A description of the problem can be found here:
///   http://en.wikipedia.org/wiki/Travelling_salesperson_problem.
/// </summary>
public class TspCircuitBoard
{
    class DataModel
    {
        public int[,] Locations = {
            { 288, 149 }, { 288, 129 }, { 270, 133 }, { 256, 141 }, { 256, 157 }, { 246, 157 }, { 236, 169 },
            { 228, 169 }, { 228, 161 }, { 220, 169 }, { 212, 169 }, { 204, 169 }, { 196, 169 }, { 188, 169 },
            { 196, 161 }, { 188, 145 }, { 172, 145 }, { 164, 145 }, { 156, 145 }, { 148, 145 }, { 140, 145 },
            { 148, 169 }, { 164, 169 }, { 172, 169 }, { 156, 169 }, { 140, 169 }, { 132, 169 }, { 124, 169 },
            { 116, 161 }, { 104, 153 }, { 104, 161 }, { 104, 169 }, { 90, 165 },  { 80, 157 },  { 64, 157 },
            { 64, 165 },  { 56, 169 },  { 56, 161 },  { 56, 153 },  { 56, 145 },  { 56, 137 },  { 56, 129 },
            { 56, 121 },  { 40, 121 },  { 40, 129 },  { 40, 137 },  { 40, 145 },  { 40, 153 },  { 40, 161 },
            { 40, 169 },  { 32, 169 },  { 32, 161 },  { 32, 153 },  { 32, 145 },  { 32, 137 },  { 32, 129 },
            { 32, 121 },  { 32, 113 },  { 40, 113 },  { 56, 113 },  { 56, 105 },  { 48, 99 },   { 40, 99 },
            { 32, 97 },   { 32, 89 },   { 24, 89 },   { 16, 97 },   { 16, 109 },  { 8, 109 },   { 8, 97 },
            { 8, 89 },    { 8, 81 },    { 8, 73 },    { 8, 65 },    { 8, 57 },    { 16, 57 },   { 8, 49 },
            { 8, 41 },    { 24, 45 },   { 32, 41 },   { 32, 49 },   { 32, 57 },   { 32, 65 },   { 32, 73 },
            { 32, 81 },   { 40, 83 },   { 40, 73 },   { 40, 63 },   { 40, 51 },   { 44, 43 },   { 44, 35 },
            { 44, 27 },   { 32, 25 },   { 24, 25 },   { 16, 25 },   { 16, 17 },   { 24, 17 },   { 32, 17 },
            { 44, 11 },   { 56, 9 },    { 56, 17 },   { 56, 25 },   { 56, 33 },   { 56, 41 },   { 64, 41 },
            { 72, 41 },   { 72, 49 },   { 56, 49 },   { 48, 51 },   { 56, 57 },   { 56, 65 },   { 48, 63 },
            { 48, 73 },   { 56, 73 },   { 56, 81 },   { 48, 83 },   { 56, 89 },   { 56, 97 },   { 104, 97 },
            { 104, 105 }, { 104, 113 }, { 104, 121 }, { 104, 129 }, { 104, 137 }, { 104, 145 }, { 116, 145 },
            { 124, 145 }, { 132, 145 }, { 132, 137 }, { 140, 137 }, { 148, 137 }, { 156, 137 }, { 164, 137 },
            { 172, 125 }, { 172, 117 }, { 172, 109 }, { 172, 101 }, { 172, 93 },  { 172, 85 },  { 180, 85 },
            { 180, 77 },  { 180, 69 },  { 180, 61 },  { 180, 53 },  { 172, 53 },  { 172, 61 },  { 172, 69 },
            { 172, 77 },  { 164, 81 },  { 148, 85 },  { 124, 85 },  { 124, 93 },  { 124, 109 }, { 124, 125 },
            { 124, 117 }, { 124, 101 }, { 104, 89 },  { 104, 81 },  { 104, 73 },  { 104, 65 },  { 104, 49 },
            { 104, 41 },  { 104, 33 },  { 104, 25 },  { 104, 17 },  { 92, 9 },    { 80, 9 },    { 72, 9 },
            { 64, 21 },   { 72, 25 },   { 80, 25 },   { 80, 25 },   { 80, 41 },   { 88, 49 },   { 104, 57 },
            { 124, 69 },  { 124, 77 },  { 132, 81 },  { 140, 65 },  { 132, 61 },  { 124, 61 },  { 124, 53 },
            { 124, 45 },  { 124, 37 },  { 124, 29 },  { 132, 21 },  { 124, 21 },  { 120, 9 },   { 128, 9 },
            { 136, 9 },   { 148, 9 },   { 162, 9 },   { 156, 25 },  { 172, 21 },  { 180, 21 },  { 180, 29 },
            { 172, 29 },  { 172, 37 },  { 172, 45 },  { 180, 45 },  { 180, 37 },  { 188, 41 },  { 196, 49 },
            { 204, 57 },  { 212, 65 },  { 220, 73 },  { 228, 69 },  { 228, 77 },  { 236, 77 },  { 236, 69 },
            { 236, 61 },  { 228, 61 },  { 228, 53 },  { 236, 53 },  { 236, 45 },  { 228, 45 },  { 228, 37 },
            { 236, 37 },  { 236, 29 },  { 228, 29 },  { 228, 21 },  { 236, 21 },  { 252, 21 },  { 260, 29 },
            { 260, 37 },  { 260, 45 },  { 260, 53 },  { 260, 61 },  { 260, 69 },  { 260, 77 },  { 276, 77 },
            { 276, 69 },  { 276, 61 },  { 276, 53 },  { 284, 53 },  { 284, 61 },  { 284, 69 },  { 284, 77 },
            { 284, 85 },  { 284, 93 },  { 284, 101 }, { 288, 109 }, { 280, 109 }, { 276, 101 }, { 276, 93 },
            { 276, 85 },  { 268, 97 },  { 260, 109 }, { 252, 101 }, { 260, 93 },  { 260, 85 },  { 236, 85 },
            { 228, 85 },  { 228, 93 },  { 236, 93 },  { 236, 101 }, { 228, 101 }, { 228, 109 }, { 228, 117 },
            { 228, 125 }, { 220, 125 }, { 212, 117 }, { 204, 109 }, { 196, 101 }, { 188, 93 },  { 180, 93 },
            { 180, 101 }, { 180, 109 }, { 180, 117 }, { 180, 125 }, { 196, 145 }, { 204, 145 }, { 212, 145 },
            { 220, 145 }, { 228, 145 }, { 236, 145 }, { 246, 141 }, { 252, 125 }, { 260, 129 }, { 280, 133 },
        };
        public int VehicleNumber = 1;
        public int Depot = 0;
    };

    /// <summary>
    ///   Euclidean distance implemented as a callback. It uses an array of
    ///   positions and computes the Euclidean distance between the two
    ///   positions of two different indices.
    /// </summary>
    static long[,] ComputeEuclideanDistanceMatrix(in int[,] locations)
    {
        // Calculate the distance matrix using Euclidean distance.
        int locationNumber = locations.GetLength(0);
        long[,] distanceMatrix = new long[locationNumber, locationNumber];
        for (int fromNode = 0; fromNode < locationNumber; fromNode++)
        {
            for (int toNode = 0; toNode < locationNumber; toNode++)
            {
                if (fromNode == toNode)
                    distanceMatrix[fromNode, toNode] = 0;
                else
                    distanceMatrix[fromNode, toNode] =
                        (long)Math.Sqrt(Math.Pow(locations[toNode, 0] - locations[fromNode, 0], 2) +
                                        Math.Pow(locations[toNode, 1] - locations[fromNode, 1], 2));
            }
        }
        return distanceMatrix;
    }

    /// <summary>
    ///   Print the solution.
    /// </summary>
    static void PrintSolution(in RoutingModel routing, in RoutingIndexManager manager, in Assignment solution)
    {
        Console.WriteLine("Objective: {0}", solution.ObjectiveValue());
        // Inspect solution.
        Console.WriteLine("Route:");
        long routeDistance = 0;
        var index = routing.Start(0);
        while (routing.IsEnd(index) == false)
        {
            Console.Write("{0} -> ", manager.IndexToNode((int)index));
            var previousIndex = index;
            index = solution.Value(routing.NextVar(index));
            routeDistance += routing.GetArcCostForVehicle(previousIndex, index, 0);
        }
        Console.WriteLine("{0}", manager.IndexToNode((int)index));
        Console.WriteLine("Route distance: {0}m", routeDistance);
    }

    public static void Main(String[] args)
    {
        // Instantiate the data problem.
        DataModel data = new DataModel();

        // Create Routing Index Manager
        RoutingIndexManager manager =
            new RoutingIndexManager(data.Locations.GetLength(0), data.VehicleNumber, data.Depot);

        // Create Routing Model.
        RoutingModel routing = new RoutingModel(manager);

        // Define cost of each arc.
        long[,] distanceMatrix = ComputeEuclideanDistanceMatrix(data.Locations);
        int transitCallbackIndex = routing.RegisterTransitCallback((long fromIndex, long toIndex) =>
                                                                   {
                                                                       // Convert from routing variable Index to
                                                                       // distance matrix NodeIndex.
                                                                       var fromNode = manager.IndexToNode(fromIndex);
                                                                       var toNode = manager.IndexToNode(toIndex);
                                                                       return distanceMatrix[fromNode, toNode];
                                                                   });

        routing.SetArcCostEvaluatorOfAllVehicles(transitCallbackIndex);

        // Setting first solution heuristic.
        RoutingSearchParameters searchParameters =
            operations_research_constraint_solver.DefaultRoutingSearchParameters();
        searchParameters.FirstSolutionStrategy = FirstSolutionStrategy.Types.Value.PathCheapestArc;

        // Solve the problem.
        Assignment solution = routing.SolveWithParameters(searchParameters);

        // Print solution on console.
        PrintSolution(routing, manager, solution);
    }
}

שינוי אסטרטגיית החיפוש

פותר הניתוב לא תמיד מחזיר את הפתרון הטוב ביותר ל-TSP, כי בעיות ניתוב מסובכות באופן חישובי. למשל, הפתרון שהוחזר בדוגמה הקודמת אינו המסלול האופטימלי.

כדי למצוא פתרון טוב יותר, תוכלו להשתמש בשיטת חיפוש מתקדמת יותר, שנקראת חיפוש מודרך מקומי, שמאפשרת לפותר הבעיות להימנע ממינימום מקומי – פתרון קצר יותר מכל המסלולים בסביבה, אבל שהוא לא המינימום הגלובלי. לאחר היציאה מהמינימום המקומי, פותר הבעיות ממשיך את החיפוש.

בדוגמאות הבאות אפשר לראות איך מגדירים חיפוש מקומי מודרך לדוגמה של הלוח.

Python

search_parameters = pywrapcp.DefaultRoutingSearchParameters()
search_parameters.local_search_metaheuristic = (
    routing_enums_pb2.LocalSearchMetaheuristic.GUIDED_LOCAL_SEARCH)
search_parameters.time_limit.seconds = 30
search_parameters.log_search = True

C++‎

RoutingSearchParameters searchParameters = DefaultRoutingSearchParameters();
searchParameters.set_local_search_metaheuristic(
    LocalSearchMetaheuristic::GUIDED_LOCAL_SEARCH);
searchParameters.mutable_time_limit()->set_seconds(30);
search_parameters.set_log_search(true);

Java

מוסיפים את הצהרת ה-'Import' הבאה בתחילת התוכנית:

import com.google.protobuf.Duration;

לאחר מכן מגדירים את הפרמטרים לחיפוש באופן הבא:

RoutingSearchParameters searchParameters =
        main.defaultRoutingSearchParameters()
            .toBuilder()
            .setFirstSolutionStrategy(FirstSolutionStrategy.Value.PATH_CHEAPEST_ARC)
            .setLocalSearchMetaheuristic(LocalSearchMetaheuristic.Value.GUIDED_LOCAL_SEARCH)
            .setTimeLimit(Duration.newBuilder().setSeconds(30).build())
            .setLogSearch(true)
            .build();

C#‎

מוסיפים את השורה הבאה בתחילת התוכנית:

using Google.Protobuf.WellKnownTypes; // Duration

לאחר מכן מגדירים את הפרמטרים לחיפוש באופן הבא:

RoutingSearchParameters searchParameters =
      operations_research_constraint_solver.DefaultRoutingSearchParameters();
    searchParameters.FirstSolutionStrategy = FirstSolutionStrategy.Types.Value.PathCheapestArc;
    searchParameters.LocalSearchMetaheuristic = LocalSearchMetaheuristic.Types.Value.GuidedLocalSearch;
    searchParameters.TimeLimit = new Duration { Seconds = 30 };
    searchParameters.LogSearch = true;

הערה: כשמשתמשים ב-GUIDED_LOCAL_SEARCH או במטא-נתונים אחרים, צריך להגדיר מגבלת זמן לפותר – אחרת, פותר הבעיות לא יסתיים. בקוד הקודם מוגדרת מגבלת זמן של 30 שניות.

למידע על שיטות מקומיות נוספות לחיפוש, קראו את המאמר אפשרויות חיפוש מקומי.

הדוגמאות שלמעלה מאפשרות גם רישום ביומן של החיפוש. אמנם אין צורך לתעד את הרישום, אבל הוא יכול לעזור בניפוי באגים.

כשמפעילים את התוכנית אחרי השינויים שצוינו למעלה, הפתרון הבא הוא קצר יותר מהפתרון שמוצג בקטע הקודם.

Objective: 2672
Route:

0 -> 3 -> 276 -> 4 -> 5 -> 6 -> 8 -> 7 -> 9 -> 10 -> 11 -> 14 -> 12 -> 13 -> 23 -> 22 -> 24 -> 21 ->
25 -> 26 -> 27 -> 28 -> 125 -> 126 -> 127 -> 20 -> 19 -> 130 -> 129 -> 128 -> 153 -> 154 -> 152 ->
155 -> 151 -> 150 -> 177 -> 176 -> 175 -> 180 -> 161 -> 160 -> 174 -> 159 -> 158 -> 157 -> 156 ->
118 -> 119 -> 120 -> 121 -> 122 -> 123 -> 124 -> 29 -> 30 -> 31 -> 32 -> 33 -> 34 -> 35 -> 36 ->
37 -> 38 -> 39 -> 40 -> 41 -> 42 -> 59 -> 60 -> 58 -> 43 -> 44 -> 45 -> 46 -> 47 -> 48 -> 49 ->
50 -> 51 -> 52 -> 53 -> 54 -> 55 -> 56 -> 57 -> 67 -> 68 -> 66 -> 69 -> 70 -> 71 -> 72 -> 73 ->
75 -> 74 -> 76 -> 77 -> 78 -> 80 -> 81 -> 88 -> 79 -> 92 -> 93 -> 94 -> 95 -> 96 -> 97 -> 98 ->
99 -> 100 -> 101 -> 102 -> 91 -> 90 -> 89 -> 108 -> 111 -> 87 -> 82 -> 83 -> 86 -> 112 -> 115 ->
85 -> 84 -> 64 -> 65 -> 63 -> 62 -> 61 -> 117 -> 116 -> 114 -> 113 -> 110 -> 109 -> 107 -> 103 ->
104 -> 105 -> 106 -> 173 -> 172 -> 171 -> 170 -> 169 -> 168 -> 167 -> 166 -> 165 -> 164 -> 163 ->
162 -> 187 -> 188 -> 189 -> 190 -> 191 -> 192 -> 185 -> 186 -> 184 -> 183 -> 182 -> 181 -> 179 ->
178 -> 149 -> 148 -> 138 -> 137 -> 136 -> 266 -> 267 -> 135 -> 134 -> 268 -> 269 -> 133 -> 132 ->
131 -> 18 -> 17 -> 16 -> 15 -> 270 -> 271 -> 272 -> 273 -> 274 -> 275 -> 259 -> 258 -> 260 -> 261 ->
262 -> 263 -> 264 -> 265 -> 139 -> 140 -> 147 -> 146 -> 141 -> 142 -> 145 -> 144 -> 198 -> 197 ->
196 -> 193 -> 194 -> 195 -> 200 -> 201 -> 199 -> 143 -> 202 -> 203 -> 204 -> 205 -> 206 -> 207 ->
252 -> 253 -> 256 -> 257 -> 255 -> 254 -> 251 -> 208 -> 209 -> 210 -> 211 -> 212 -> 213 -> 214 ->
215 -> 216 -> 217 -> 218 -> 219 -> 220 -> 221 -> 222 -> 223 -> 224 -> 225 -> 226 -> 227 -> 232 ->
233 -> 234 -> 235 -> 236 -> 237 -> 230 -> 231 -> 228 -> 229 -> 250 -> 245 -> 238 -> 239 -> 240 ->
241 -> 242 -> 243 -> 244 -> 246 -> 249 -> 248 -> 247 -> 277 -> 278 -> 2 -> 279 -> 1 -> 0

אפשרויות חיפוש נוספות מפורטות במאמר אפשרויות מסלול.

האלגוריתמים הטובים ביותר יכולים עכשיו לפתור באופן קבוע מופעים של TSP עם עשרות אלפי צמתים. (הרשומה בזמן הכתיבה היא המכונה pla85900 ב-TSPLIB, אפליקציית VLSI עם 85,900 צמתים. במקרים מסוימים עם מיליוני צמתים, נמצא שפתרונות מסוימים נמצאים בטווח של 1% מהסיור האופטימלי.)

שינוי גודל מטריצת המרחק

מאחר שפותר הניתוב פועל על כל המספרים השלמים, אם מטריצת המרחקים מכילה ערכים לא שלמים, תצטרכו לעגל את המרחקים למספרים שלמים. אם מרחקים מסוימים קטנים, עיגול הנתונים עלול להשפיע על הפתרון.

כדי להימנע מבעיות בעיגול, אפשר scale את מטריצת המרחק: מכפילים את כל הערכים של המטריצה במספר גדול, למשל 100. הפעולה הזו מכפילה את האורך של כל מסלול בפקטור של 100, אבל לא משנה את הפתרון. היתרון הוא שמעכשיו, כאשר מעוגלים את ערכי המטריצה, כמות העיגולים (שהיא לכל היותר 0.5) קטנה מאוד בהשוואה למרחקים, כך שהיא לא משפיעה באופן משמעותי על הפתרון.

אם משנים את קנה המידה של מטריצת המרחק, צריך לשנות את המדפסת לפתרון כדי לחלק את האורכים של הנתיבים לפי קנה מידה, לפי קנה המידה שלה, כדי להציג את המרחקים שאינם מותאמים למסלולים.